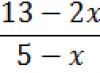Surface waves on the water. Waves on the surface of the water

WAVES ON THE SURFACE OF A LIQUID- wave movements of a liquid, the existence of which is associated with a change in the shape of its boundary. Naib. An important example is waves on the free surface of a body of water (ocean, sea, lake, etc.), formed due to the action of gravity and surface tension. If s-l. ext. impact (a thrown stone, the movement of a ship, a gust of wind, etc.) disturbs the equilibrium of the liquid, then these forces, trying to restore balance, create movements transmitted from one particle of the liquid to another, generating waves. In this case, the wave movements cover, strictly speaking, the entire thickness of the water, but if the depth of the reservoir is large compared to the wavelength, then these movements are concentrated. arr. in the near-surface layer, practically not reaching the bottom (short waves, or waves in deep water). The simplest type of such waves is a plane sinusoidal wave, in which the surface of the liquid is sinusoidally “corrugated” in one direction, and all disturbances are physical. quantities, for example vertical particle displacements have the form where X- horizontal, z - vertical coordinates, - angular. frequency, k- wave number, A- amplitude of particle vibrations, depending on depth z. Solving the equations of hydrodynamics of an incompressible fluid together with boundary conditions (constant pressure on the surface and absence of disturbances at great depth) shows that , Where A 0- amplitude of surface displacement. In this case, each particle of liquid moves in a circle, the radius of which is equal to A(z) (Fig., a). Thus, the oscillations decay exponentially deep into the liquid, and the faster the shorter the wave (the longer k). Quantities are related dispersion equation

where is the density of the liquid, g- free fall acceleration, - coefficient. surface tension. From this formula the phase velocity is determined, with which a fixed point moves. phase (for example, the top of a wave), and group velocity is the speed of energy movement. Both of these speeds, depending on k(or wavelength ) have a minimum; yes, min. the value of the phase velocity of waves in clean water (devoid of polluting films that affect surface tension) is achieved at 1.7 cm and is equal to 23 cm/c. Waves of much shorter length are called. capillary, and longer ones - gravitational, since there are advantages to their distribution. the influence is exerted respectively by the forces of surface tension and gravity. For purely gravitational waves . In the mixed case they speak of gravitational-capillary waves.

Trajectories of movement of water particles in a sinusoidal wave: a - in deep water, b - in shallow water.

In general, the characteristics of waves are affected by the total depth of the liquid H. If vertical. the displacement of the liquid at the bottom is zero (hard bottom), then in a plane sinusoidal wave the amplitude of oscillations changes according to the law: , and dispersion. The level of waves in a reservoir of finite depth (without taking into account the rotation of the Earth) has the form

For short waves, this equation coincides with (1). For long waves, or waves on shallow water, if the effects of capillarity can be neglected (for long waves they are usually significant only in the case of thin films of liquid), it takes the form In such a wave, the phase and group velocities are equal to the same value, independent of frequency . This speed value is the highest for gravity. waves in a given body of water; in the deepest place of the ocean ( H=11 km) it is 330 m/s. The movement of particles in a long wave occurs along ellipses that are strongly elongated in the horizontal direction, and the amplitude of the horizontal movements of particles is almost the same throughout the entire depth (Fig. b).

The listed properties are possessed only by waves of sufficiently small amplitude (much less than both the wavelength and the depth of the reservoir). Intense nonlinear waves have a substantially non-sinusoidal shape, depending on the amplitude. The nature of the nonlinear process depends on the relationship between the wavelength and the depth of the reservoir. Short gravitational waves in deep water acquire pointed peaks, which when defined. critical value of their height collapse with the formation of capillary “ripples” or foam “lambs”. Waves of moderate amplitude can have a stationary shape that does not change during propagation. According to Gerstner's theory, in a nonlinear stationary wave the particles still move in a circle, but the surface has the shape of a trochoid, the edges at low amplitude coincide with a sinusoid, and at a certain max. critical amplitude equal to , turns into a cycloid with “points” at the vertices. Results that are closer to observational data are given by the Stokes theory, according to which particles in a stationary nonlinear wave move along open trajectories, that is, they “drift” in the direction of wave propagation, and at critical. amplitude value (slightly smaller), at the top of the wave it is not a “tip” that appears, but a “kink” with an angle of 120°.

For long nonlinear waves in shallow water, the speed of movement of any point in the profile increases with height, so the top of the wave catches up with its base; As a result, the steepness of the leading wave slope continuously increases. For relatively low waves, this increase in steepness is stopped by the dispersion associated with the finite depth of the reservoir; such waves are described Korteweg-de Vries equation. Stationary waves in shallow water can be periodic or solitary (see. Soliton); for them there is also a critical height at which they collapse. To the spread of long waves of creatures. influenced by the bottom topography. Thus, approaching a gently sloping shore, the waves suddenly slow down and collapse (surf); When a wave from the sea enters the river bed, a steep foaming front - a bore - can form, moving up the river in the form of a sheer wall. Tsunami waves in the area of the source of the earthquake that excites them are almost imperceptible, but when they reach a relatively shallow coastal area - the shelf, they sometimes reach great heights, posing a formidable danger to coastal settlements.

In real conditions, V. on p.zh. are not flat, but have a more complex spatial structure, depending on the characteristics of their source. For example, a stone falling into water generates circular waves (see. Cylindrical wave).The movement of the vessel excites ship waves; one system of such waves diverges from the bow of the vessel in the form of a “whisker” (in deep water, the angle between the “whiskers” does not depend on the speed of the source and is close to 39°), the other moves behind its stern in the direction of the ship’s movement. The sources of long waves in the ocean are the gravitational forces of the Moon and the Sun, which generate tides, as well as underwater earthquakes and volcanic eruptions - the sources of tsunami waves.

Wind waves have a complex structure, the characteristics of which are determined by the speed of the wind and the time of its influence on the wave. The mechanism of energy transfer from wind to wave is due to the fact that pressure pulsations in the air flow deform the surface. In turn, these deformations affect the distribution of air pressure near the water surface, and these two effects can reinforce each other, and as a result, the amplitude of surface disturbances increases (see Fig. Self-oscillations). In this case, the phase speed of the excited wave is close to the wind speed; Thanks to this synchronism, air pulsations act “in time” with the alternation of elevations and depressions (resonance in time and space). This condition can be satisfied for waves of different frequencies traveling in different directions. directions relative to the wind; The energy they receive is then partially transferred to other waves due to nonlinear interactions (see. Waves). As a result, developed waves are a random process characterized by a continuous distribution of energy in frequencies and directions (spatio-temporal spectrum). Waves leaving the area of the wind (swell) take on a more regular shape.

Waves similar to waves on a liquid line also exist at the interface between two immiscible liquids (see. Internal waves).

In the ocean, waves are studied. methods using waveographs that monitor fluctuations in the water surface, as well as remote control. methods (photography of the sea surface, use of radio and sonar) - from ships, aircraft and satellites.

Lit.: Bascom W., Waves and Beaches, [trans. from English], L., 1966; Trikker R., Bor, surf, waves and ship waves, [trans. from English], L., 1969; Whitham J., Linear and nonlinear waves, trans. from English, M., 1977; Physics of the ocean, vol. 2 - Hydrodynamics of the ocean, M., 1978; Kadomtsev B.B., Rydnik V.I., Waves around us, M., 1981; Lighthill J., Waves in Liquids, trans. from English, M., 1981; Le Blon P., Majsek L., Waves in the Ocean, trans. from English, [part] 1-2, M., 1981. L. A. Ostrovsky.

The formulas derived above are only suitable for waves in deep water. They are still quite accurate if the water depth is equal to half the wavelength. At shallower depths, water particles on the surface of the wave describe not circular trajectories, but elliptical ones, and the derived relationships are incorrect and actually take on a more complex form. However, for waves in very shallow water, as well as for very long waves in medium water, the relationship between the length and speed of wave propagation again takes a simpler form. In both of these cases, the vertical movements of water particles on the free surface are very small compared to the horizontal movements. Therefore, again we can assume that the waves have an approximately sinusoidal shape. Since the particle trajectories are very flattened ellipses, the effect of vertical acceleration on the pressure distribution can be neglected. Then at each vertical the pressure will change according to a static law.

Let a “shaft” of water of width b spread at a speed c from right to left on the water surface above a flat bottom, increasing the water level from h 1 to h 2 (Figure 4.4). Before the arrival of the swell, the water was at rest. The speed of her movement after increasing the level of shield. This speed does not coincide with the speed of the shaft; it is necessary in order to cause a lateral movement of the volume of water in the transition zone of width b to the right and thereby raise the water level.

Fig 4.4 n

The inclination of the shaft over its entire width is assumed to be constant and equal. Provided that the speed u is small enough that it can be neglected in comparison with the speed c of the propagation of the shaft, the vertical speed of water in the area of the shaft will be equal to (Figure 4.5)

Continuity condition 3.4, applied to a single layer of water (in the direction perpendicular to the plane of Figure 4.4), has the form

u 1 l 1 = u 2 l 2 , (the integral disappeared due to the linearity of the areas under consideration),

here u 1 and u 2 are the average velocities in the cross sections l 1 and l 2 of the flow, respectively. l 1 and l 2 - linear quantities (lengths).

This equation, applied to this case, leads to the relation

h 2 u = bV, or h 2 u = c (h 2 -h 1). (4.9)

From 4.9 it is clear that the relationship between the speeds u and c does not depend on the width of the shaft.

Equation 4.9 remains true for a shaft of a non-rectilinear profile (provided that the angle b is small). This is easy to show by dividing such a shaft into a number of narrow shafts with straight profiles and adding up the continuity equations compiled for each individual shaft:

Where, provided that the difference h 2 - h 1 can be neglected and instead of h 2i in each case, substitute h 2, it turns out. This condition is valid under the already accepted assumption that the velocity u is small (see 4.9).

To the kinematic relation 4.9 should be added a dynamic relation derived from the following considerations:

A volume of water with width b in the area of the shaft is in accelerated motion, since the particles that make up this volume begin their movement on the right edge with zero speed, and on the left edge they have speeds w (Figure 4.4). An arbitrary particle of water is taken from the area inside the shaft. The time it takes for the shaft to pass over this particle is

therefore the particle acceleration

Next, the width of the shaft (its linear dimension in a plane perpendicular to the figure) is taken equal to one (Figure 4.6). This allows us to write the expression for the mass of the volume of water located in the shaft area as follows:

Where h m is the average water level in the shaft area. (4.11)

The pressure difference on both sides of the shaft at the same height is (according to the hydrostatic formula) , where is a constant for a given substance (water).

Therefore, the total pressure force acting on the considered volume of water in the horizontal direction is equal. Newton's second law (the basic equation of dynamics), taking into account 4.10 and 4.11, will be written as:

Where. (4.12)

So the shaft width was taken out of the equation. In the same way as was done for equation 4.9, it is proven that equation 4.12 is also applicable for a shaft with a different profile, provided that the difference h 2 - h 1 is small compared to h 2 and h 1 themselves.

So, there is a system of equations 4.9 and 4.12. Next, on the left side of equation 4.9, h 2 is replaced by h m (which, with a low shaft and, as a consequence, a small difference h 2 - h 1, is quite acceptable) and equation 4.12 is divided into equation 4.9:

After the reductions it turns out

The alternation of shafts with symmetrical angles of inclination (the so-called positive and negative shafts) leads to the formation of waves. The speed of propagation of such waves does not depend on their shape.

Long waves in shallow water travel at a speed called the critical speed.

If several low shafts follow each other on the water, each of which slightly increases the water level, then the speed of each subsequent shaft is slightly greater than the speed of the previous shaft, since the latter has already caused a slight increase in the depth h. In addition, each subsequent shaft no longer propagates in still water, but in water already moving in the direction of movement of the shaft at a speed of All this leads to the fact that subsequent shafts catch up with the previous ones, resulting in a steep shaft of finite height.

Which decreases with distance from the surface. Waves on the surface of a liquid can fill large areas, consist of several waves (train) and even one crest or trough (solitary wave, soliton). The periods of waves on the surface of a liquid range from several days to fractions of a second, lengths from thousands of kilometers to fractions of a millimeter, amplitudes from tens of meters to fractions of a micrometer. The wave type, phase and group velocities are specified by the dispersion relation ω = ω(k) - a function of frequency ω on the wave vector k. The lowest frequency waves on the surface of a liquid - inertial waves - are caused by the Coriolis force; intermediate frequency waves - gravitational waves on the surface of a liquid - gravity with acceleration g. Short and high-frequency waves on the surface of a liquid - capillary waves - are created by surface tension forces. For short gravitational waves on the surface of a liquid (λ< 5Н, где λ = 2π/k - длина волны, Н - глубина водоёма) фазовая скорость больше групповой и растёт с длиной волны (прямая дисперсия). Частицы в них описывают окружности, радиус которых убывает с глубиной. Скорость длинных волн на поверхности жидкости (λ>10H) does not depend on λ (waves without dispersion); the particles in them move along ellipses with a decreasing vertical axis. Capillary waves on the surface of a liquid have inverse dispersion; their group velocity is greater than the phase velocity. Fast capillary waves on the surface of a liquid are located in front of the obstacle, slow gravitational waves are located behind it. The speed of the slowest waves on the surface of a liquid determines the size of the area of quiet water that separates the train of non-stationary waves from a pulsed source, for example, a stone thrown into the water. Near the surface of a viscous fluid, waves form a periodic boundary layer with thickness δ = √2 ν/ω, where V is the kinematic viscosity. Waves on the surface of a liquid and accompanying boundary layers transport energy and matter.

The picture of waves on the surface of a liquid is complicated by wave interference (superposition of waves from various sources), reflection (reflection from uneven bottoms and shores), refraction (curvature and rotation of wave fronts on an uneven bottom), diffraction (penetration into the region of a geometric shadow), as well as nonlinear interaction with waves on the surface and inside the liquid, boundary layers, currents, vortices and wind. As the amplitude increases, the differences in the properties of the wave and the boundary layer are erased, and a single wave-vortex system (“boiling wall of water”, “rogue wave”) is formed, which has great destructive power. Waves on the surface of a liquid disintegrate if the acceleration in them exceeds g and the amplitude A >λ/2π.

Waves on the surface of liquid in the oceans are formed under the influence of the attraction of the Moon and the Sun (the most pronounced are tidal waves with periods that are multiples of 12 hours 25 minutes - half a lunar day), earthquakes and landslides that change the shape of the bottom and shores (tsunamis with a period of 10-30 minutes) , due to the influence of the atmosphere, flow around obstacles. Wind waves with a period of 2-16 s propagate at a speed of 3-25 m/s over long distances, forming regular swell and surf. The amplitude of tsunamis, traveling in the ocean at a speed of about 700 km/h, increases as they approach the coast; they wash away cities and devastate coastal areas.

Waves on the surface of a liquid affect the exchange of matter, energy and momentum between the atmosphere and the hydrosphere, and contribute to the saturation of water with oxygen. The renewable energy of waves on the surface of the liquid is used by tidal power plants and installations that directly convert it into electricity.

See what “Waves on the surface of a liquid” are in other dictionaries:

Waves that arise and propagate along the free surface of a liquid or along the interface between two immiscible liquids. V. on p.zh. are formed under the influence of external influences. impact, as a result of which the surface of the liquid is removed from... ... Physical encyclopedia

Continuum mechanics ... Wikipedia

Wave movements of the boundary of a liquid (for example, the surface of the ocean) that occur when the equilibrium of the liquid is disturbed (by the action of wind, a passing ship, a thrown stone) and the tendency of gravity and surface tension forces of the liquid... ... Natural science. Encyclopedic Dictionary

Waves on the surface of the sea or ocean. Due to their high mobility, water particles, under the influence of various kinds of forces, easily leave the state of equilibrium and perform oscillatory movements. The reasons that cause waves to appear are... ... Great Soviet Encyclopedia

Changes in the state of the environment (disturbances) that propagate in this environment and carry with them energy. The most important and common types of waves are elastic waves, waves on the surface of a liquid, and electromagnetic waves. Special cases of elastic V.... ... Physical encyclopedia

Waves- Waves: a single wave; b wave train; c infinite sine wave; l wavelength. WAVES, changes in the state of a medium (disturbances) propagating in this medium and carrying energy with them. The main property of all waves, regardless of their... ... Illustrated Encyclopedic Dictionary

Disturbances that propagate at a finite speed in space and carry with them energy without transferring matter. The most common are elastic waves, such as sound waves, waves on the surface of a liquid and electromagnetic waves. Despite… … Big Encyclopedic Dictionary

Continuum mechanics Continuum Classical mechanics Law of conservation of mass Law of conservation of momentum ... Wikipedia

A wave is a change in the state of a medium (perturbation) that propagates in this medium and carries energy with it. In other words: “...waves or waves are the spatial alternation of maxima and minima of any... ... Wikipedia that changes over time

Disturbances that propagate at a finite speed in space and carry with them energy without transferring matter. The most common types of waves are elastic waves, such as sound waves, waves at the surface of liquids, and electromagnetic waves. Despite… … Encyclopedic Dictionary

Books

Dynamics of multiphase systems. Study guide, Glazkov Vasily Valentinovich. The course "Dynamics of Multiphase Systems" is a continuation of the main course on heat and mass transfer. The course formulates a mathematical description and models of two-phase systems. Are being considered...

Waves formed on the free surface of the water set the air in contact with them in motion. In most cases, the mass of this air can be neglected compared to the mass of the liquid. Then the pressure on the free surface of the liquid will be equal to atmospheric pressure. Observations show that with the simplest wave motion, individual particles of the free surface of water describe trajectories that approximately coincide with a circle. In a reference frame moving along with the waves at the speed of their propagation, the wave motion is obviously a steady motion (Fig. 80). Let the speed of wave propagation be equal to c, the radius of the circle described by a particle of water located on a free surface be equal, and the period of revolution of this particle along its trajectory be equal to Then in the indicated reference system the speed of the current at the wave crests will be equal to

and in the troughs of the waves

Since the difference in height between the highest and lowest positions of the points on the free surface is equal, applying Bernoulli's equation to the streamline located on the free surface, we obtain:

or, after substituting instead and their values,

whence it follows that

The radius is not included in this formula, therefore, the speed of wave propagation does not depend on the height of the waves. When waves propagate, the wave crest moves over time through a distance called the wavelength, therefore,

Eliminating the period from equalities (60) and (61), we obtain:

Thus, for waves on the surface of water, their speed of propagation, unlike sound waves, strongly depends on the wavelength. Long waves travel faster than short ones. Waves of different lengths can overlap each other without noticeable mutual disturbance. In this case, the short waves seem to be lifted by the long waves, but then the long waves move forward, and the short waves remain behind them. Streamlines in a reference frame stationary relative to undisturbed water are shown in Fig. 81. From the location of the streamlines it is clear that the speed of water decreases very quickly with increasing depth, namely, in proportion to the decrease in value; therefore, at a depth equal to the wavelength, the speed is only the speed on the free surface.

Rice. 81. Streamlines of wave motion

The exact theory shows that formula (62) is valid only for low waves, regardless of their height. For high waves, the speed c is actually somewhat greater than the value given by formula (62). In addition, with high waves, the trajectories of water particles located on the free surface are not closed: the water at the crest of the wave goes forward a greater distance than the distance to which it returns back in the trough of the wave (see the right side of Fig. 81). Consequently, with high waves, water is transferred forward.

For waves with a short length, an important factor is, in addition to gravity, surface tension. It tends to smooth out the wave surface, and therefore the speed of wave propagation increases. Theory shows that in this case the speed of wave propagation is equal to

where C is the capillary constant. For long waves, the predominant role is played by the first term under the root sign, and for short waves, on the contrary, the second term. For wavelength

the propagation speed c has a minimum value equal to

For water dynes/cm, therefore,

Waves whose length is longer are called gravitational, and waves whose length is shorter are called capillary.

The speed of propagation of the group should be distinguished from the speed of movement of the wave crests, called the phase speed (we called it the speed of wave propagation above and denoted it by c).

waves, called group velocity and denoted by c. The easiest way to explain the meaning of this concept is by the example of movement resulting from the superposition of two waves having equal amplitudes, but slightly different in length. Let us have a sine wave

where A is amplitude, time, and some coefficients. When increasing by y or y, the sine takes on the same value, therefore, the quantity

is the wavelength, and the magnitude

there is a period of oscillation. If

i.e. if

then the argument of the sine does not depend on time, therefore the ordinate y does not depend on time. This means that the entire wave, without changing its shape, moves to the right with a speed

Let's superimpose a second wave on this wave

i.e. a wave with the same amplitude A, but with slightly different values. The resulting movement will be

At those points of the x axis at which the phases of both oscillations coincide, the amplitude is equal at the same points at which the phases of both oscillations

are opposite, the amplitude is zero. This phenomenon is called beating. Applying the well-known formula

we will get:

In this equality the term

represents a wave for which the coefficients are equal to the average values of and, respectively, the Multiplier

which changes slowly for small values of the differences, can be considered as a variable amplitude (Fig. 82).

Rice. 82. Beat

The wave group ends at the point where the cosine becomes zero. The speed of movement of this point, called the group velocity c, based on considerations similar to the previous ones, is equal to

For long groups, i.e. for slow beats, with sufficient accuracy we can assume that

For waves arising under the influence of gravity, from formula (60) we have:

But, according to equality (65),

hence,

On the other hand, substituting the value from equality (64) into formula (62), we get:

From here, differentiating with respect to and bearing in mind equality (67), we find:

Thus, groups of waves travel at a speed c equal to half the phase speed, in other words, the crests in a group of waves move at twice the speed of the group of waves; At the back end of the group new waves appear all the time, and at the front end of the group they disappear. This phenomenon is very easy to observe in waves caused by a stone falling into still water.

All of the above applies not only to waves on the surface of water, but also to any other waves whose phase velocity depends on the wavelength.

Another type of wave group is the waves that appear on the surface of the water when a ship moves. A wave pattern very similar to ship waves can be easily obtained if a point source of pressure disturbance is made to move at a constant speed on the surface of deep, resting water. The resulting movement can be studied mathematically. According to the calculations of V. Thomson (lord Kelvin), Ekman and others, the wave system shown in Fig. is obtained. 83, on which wave crests are indicated by inclined lines. This wave system moves along with the source of disturbance. The length of transverse waves based on formula (62) is equal to

where c is the speed of movement of the source of disturbance. When a ship moves, two systems of such waves are formed - one near the bow, the other near the stern of the ship, and the waves of both systems interfere with each other.

Rice. 83. A system of waves formed during uniform movement of a source of pressure disturbance on the surface of the water

The group velocity of capillary waves, as can be easily shown by a calculation similar to that made for gravitational waves, is greater than the phase velocity, namely, in the limiting case of very small waves, by a factor of 1.5. Consequently, if the source of disturbance moves at a constant speed, then groups of waves are ahead of it. Near the line of a fishing rod lowered into a river, the flow speed of which is more than 23.3 cm/sec, capillary waves are formed upstream, and gravity waves are formed downstream, and the latter have approximately the same shape as in Fig. 83, and the first ones diverge upstream in the form of circular arcs. At speeds of movement of the source of disturbance less than 23.3 cm/sec, waves are not formed.

Waves can also appear on the contact surface of two liquids of different densities, located one above the other. If both liquids are motionless and their densities are equal, then the theoretical calculation gives the value for the phase velocity of waves

If the upper fluid flows at a speed relative to the lower one, then the theory shows that the resulting waves are stable only if their length is sufficiently large. Short waves, just as was shown in § 7 for the movement of two liquid flows along the interface, are unstable, which leads to mixing of both liquids in the intermediate zone; this mixing restores the stability of the flow. As the speed increases, the boundary between instability and stability moves towards waves with longer wavelengths. Waves of this kind can also arise in the atmosphere at the boundary of two layers of air of different densities moving relative to each other; Sometimes these waves are made visible by the formation of so-called wavy clouds.

When air moves over the surface of the water, waves are also formed. However, the theory of such waves, based on the assumption of the absence of friction, leads to results that contradict

reality. So, for example, calculations by V. Thomson showed that the minimum wind speed required for the formation of waves on the surface of the water should be a round number, and waves appear that have a minimum propagation speed cm/sec and a wavelength cm (at higher wind speeds, of course, , waves with longer length). Meanwhile, in reality, for the formation of waves, a wind with a speed is sufficient. According to Jeffrey's research, this is explained by the fact that due to friction, the pressure distribution on the surface of the wave becomes asymmetrical, and therefore the wind, if its speed is greater than the phase speed of the waves, does work on the crest of each wave. Motzfeld, by measuring the pressure distribution on the surface of model water waves, found that the resistance that air provides to the movement of waves is proportional to one and a half degrees of inclination of the wave surface at the inflection point relative to the horizon, as well as the square of the difference between the wind speed and the phase speed of the waves. Further, Motzfeld found by calculation that the inclination of the wave surface at the inflection point, depending on the phase velocity c, is greatest at

This speed c corresponds, based on formula (62), to a wave of length

If we take into account surface tension, which Motzfeld did not take into account, then the calculation shows that, in full accordance with observations, a wind with a speed slightly exceeding 23.3 cm/sec is sufficient to cause a slight disturbance on the surface of the water.

The formulas derived above are only suitable for waves in deep water. They are still quite accurate if the water depth is equal to half the wavelength. At shallower depths, water particles on the surface of the wave describe elliptical rather than circular trajectories, and the relationship between the length and speed of wave propagation is more complex than for waves in deep water. However, for waves at

in very shallow water, as well as for very long waves in medium water, the dependence just indicated again takes a simpler form. In both of the latter cases, the vertical movements of water particles on the free surface are very small compared to the horizontal movements. Therefore, we can again assume that the waves have an approximately sinusoidal shape. Since (particle trajectories are very flattened ellipses, the influence of vertical acceleration on the pressure distribution can be neglected. Then at each vertical the pressure will change according to a static law, and differences in the heights of the liquid will determine almost only horizontal accelerations. We will limit ourselves here to calculations only for the case the movement of the water “shaft” shown in Fig. 84. These calculations are very simple and will be used by us in the future to study the propagation of pressure disturbances in a compressible medium (see § 2 of Chapter IV).

Rice. 84. Shaft on the surface of the water

Suppose that on the surface of the water above a flat bottom, a shaft with a width increasing the water level from to propagates at a speed c from right to left. Let us assume that before the arrival of the shaft the water was at rest. The speed of its movement after the level rise will be denoted by This speed, which does not at all coincide with the speed c of the propagation of the shaft, is necessary in order to cause a lateral movement of the volume of water in the transition zone wide to the right and thereby raise the water level from a height to a height. Let us assume for simplicity that the inclination of the shaft over its entire width is constant, therefore, it is equal to Then, provided that the speed is small enough to be neglected in comparison with the speed c of the propagation of the shaft, the vertical speed of the rise of water in the area of the shaft will be equal to and the difference in heights must also be small therefore, this equation applies only to low shafts, and therefore the condition just mentioned is quite justified.

The kinematic relation (72) should be accompanied by a dynamic relation, which can be easily derived as follows. A volume of water with a width in the area of the shaft is in accelerated motion, since the particles that make up this volume begin their movement on the right edge with a speed of zero, and on the left edge they have velocities. Let's take some particle of water in the area of the shaft. The time during which the shaft passes over this particle is obviously equal to

therefore the acceleration of the particle will be

The volume of water in the area of the shaft, if its thickness in the direction perpendicular to the plane of the figure is taken equal to unity, has a mass where In addition, each subsequent shaft does not propagate in stationary water, but in water already moving to the right with speed. This leads to that subsequent shafts catch up with the previous ones, resulting in a steep shaft of finite height.

The study of the propagation of a shaft of finite height can be carried out using the momentum theorem in exactly the same way as was done in § 13 when considering the sudden expansion of a flow. In order for the movement of water during the propagation of the shaft to be considered as steady, the calculation should be carried out in a reference system moving along with the shaft. The speed of propagation of the shaft of final height is greater than