Two conditions for a regular pyramid by its definition. Pyramid and its elements

A three-dimensional figure that often appears in geometric problems is the pyramid. The simplest of all the figures in this class is triangular. In this article we will analyze in detail the basic formulas and properties of the correct

Geometric ideas about the figure

Before moving on to considering the properties of a regular triangular pyramid, let’s take a closer look at what kind of figure we are talking about.

Let's assume that there is an arbitrary triangle in three-dimensional space. Let us select any point in this space that does not lie in the plane of the triangle and connect it with the three vertices of the triangle. We got a triangular pyramid.

It consists of 4 sides, all of which are triangles. The points where three faces meet are called vertices. The figure also has four of them. The lines of intersection of two faces are edges. The pyramid in question has 6 edges. The figure below shows an example of this figure.

Since the figure is formed by four sides, it is also called a tetrahedron.

Correct pyramid

Above we considered an arbitrary figure with a triangular base. Now suppose that we draw a perpendicular segment from the top of the pyramid to its base. This segment is called height. Obviously, you can draw 4 different heights for the figure. If the height intersects the triangular base at the geometric center, then such a pyramid is called straight.

A straight pyramid, the base of which is an equilateral triangle, is called regular. For her, all three triangles forming the lateral surface of the figure are isosceles and equal to each other. A special case of a regular pyramid is the situation when all four sides are equilateral identical triangles.

Let's consider the properties of a regular triangular pyramid and give the corresponding formulas for calculating its parameters.

Base side, height, lateral edge and apothem

Any two of the listed parameters uniquely determine the remaining two characteristics. Let us present formulas that relate these quantities.

Let us assume that the side of the base of a regular triangular pyramid is a. The length of its lateral edge is b. What will be the height of a regular triangular pyramid and its apothem?

For height h we get the expression:

This formula follows from the Pythagorean theorem for which the side edge, the height and 2/3 of the height of the base are.

The apothem of a pyramid is the height for any side triangle. The length of the apothem a b is equal to:

a b = √(b 2 - a 2 /4)

From these formulas it is clear that whatever the side of the base of a triangular regular pyramid and the length of its side edge, the apothem will always be greater than the height of the pyramid.

The two formulas presented contain all four linear characteristics of the figure in question. Therefore, given the known two of them, you can find the rest by solving the system of written equalities.

Figure volume

For absolutely any pyramid (including an inclined one), the value of the volume of space limited by it can be determined by knowing the height of the figure and the area of ​​its base. The corresponding formula is:

Applying this expression to the figure in question, we obtain the following formula:

Where the height of a regular triangular pyramid is h and its base side is a.

It is not difficult to obtain a formula for the volume of a tetrahedron in which all sides are equal to each other and represent equilateral triangles. In this case, the volume of the figure is determined by the formula:

That is, it is determined uniquely by the length of side a.

Surface area

Let's continue to consider the triangular regular one. The total area of ​​all the faces of a figure is called its surface area. The latter can be conveniently studied by considering the corresponding development. The figure below shows what the development of a regular triangular pyramid looks like.

Let's assume that we know the height h and the side of the base a of the figure. Then the area of ​​its base will be equal to:

Every schoolchild can obtain this expression if he remembers how to find the area of ​​a triangle, and also takes into account that the altitude of an equilateral triangle is also a bisector and a median.

The lateral surface area formed by three identical isosceles triangles is:

S b = 3/2*√(a 2 /12+h 2)*a

This equality follows from the expression of the apothem of the pyramid in terms of the height and length of the base.

The total surface area of ​​the figure is:

S = S o + S b = √3/4*a 2 + 3/2*√(a 2 /12+h 2)*a

Note that for a tetrahedron in which all four sides are identical equilateral triangles, the area S will be equal to:

Properties of a regular truncated triangular pyramid

If the top of the considered triangular pyramid is cut off with a plane parallel to the base, then the remaining lower part will be called a truncated pyramid.

In the case of a triangular base, the result of the described sectioning method is a new triangle, which is also equilateral, but has a shorter side length than the side of the base. A truncated triangular pyramid is shown below.

We see that this figure is already limited by two triangular bases and three isosceles trapezoids.

Let us assume that the height of the resulting figure is equal to h, the lengths of the sides of the lower and upper bases are a 1 and a 2, respectively, and the apothem (height of the trapezoid) is equal to a b. Then the surface area of ​​the truncated pyramid can be calculated using the formula:

S = 3/2*(a 1 +a 2)*a b + √3/4*(a 1 2 + a 2 2)

Here the first term is the area of ​​the lateral surface, the second term is the area of ​​the triangular bases.

The volume of the figure is calculated as follows:

V = √3/12*h*(a 1 2 + a 2 2 + a 1 *a 2)

To unambiguously determine the characteristics of a truncated pyramid, it is necessary to know its three parameters, as demonstrated by the given formulas.

A pyramid is a polyhedron with a polygon at its base. All faces, in turn, form triangles that converge at one vertex. Pyramids are triangular, quadrangular, and so on. In order to determine which pyramid is in front of you, it is enough to count the number of angles at its base. The definition of “height of a pyramid” is very often found in geometry problems in the school curriculum. In this article we will try to look at different ways to find it.

Parts of the pyramid

Each pyramid consists of the following elements:

• side faces, which have three corners and converge at the apex;
• the apothem represents the height that descends from its apex;
• the top of the pyramid is a point that connects the side ribs, but does not lie in the plane of the base;
• the base is a polygon on which the vertex does not lie;
• the height of a pyramid is a segment that intersects the top of the pyramid and forms a right angle with its base.

How to find the height of a pyramid if its volume is known

Through the formula V = (S*h)/3 (in the formula V is the volume, S is the area of ​​the base, h is the height of the pyramid) we find that h = (3*V)/S. To consolidate the material, let's immediately solve the problem. The triangular base is 50 cm 2 , whereas its volume is 125 cm 3 . The height of the triangular pyramid is unknown, which is what we need to find. Everything is simple here: we insert the data into our formula. We get h = (3*125)/50 = 7.5 cm.

How to find the height of a pyramid if the length of the diagonal and its edges are known

As we remember, the height of the pyramid forms a right angle with its base. This means that the height, edge and half of the diagonal together form Many, of course, remember the Pythagorean theorem. Knowing two dimensions, it will not be difficult to find the third quantity. Let us recall the well-known theorem a² = b² + c², where a is the hypotenuse, and in our case the edge of the pyramid; b - the first leg or half of the diagonal and c - respectively, the second leg, or the height of the pyramid. From this formula c² = a² - b².

Now the problem: in a regular pyramid the diagonal is 20 cm, when the length of the edge is 30 cm. You need to find the height. We solve: c² = 30² - 20² = 900-400 = 500. Hence c = √ 500 = about 22.4.

How to find the height of a truncated pyramid

It is a polygon with a cross section parallel to its base. The height of a truncated pyramid is the segment that connects its two bases. The height can be found for a regular pyramid if the lengths of the diagonals of both bases, as well as the edge of the pyramid, are known. Let the diagonal of the larger base be d1, while the diagonal of the smaller base is d2, and the edge has length l. To find the height, you can lower the heights from the two upper opposite points of the diagram to its base. We see that we have two right triangles; all that remains is to find the lengths of their legs. To do this, subtract the smaller one from the larger diagonal and divide by 2. So we will find one leg: a = (d1-d2)/2. After which, according to the Pythagorean theorem, all we have to do is find the second leg, which is the height of the pyramid.

Now let's look at this whole thing in practice. We have a task ahead of us. A truncated pyramid has a square at the base, the diagonal length of the larger base is 10 cm, while the smaller one is 6 cm, and the edge is 4 cm. You need to find the height. First, we find one leg: a = (10-6)/2 = 2 cm. One leg is equal to 2 cm, and the hypotenuse is 4 cm. It turns out that the second leg or height will be equal to 16-4 = 12, that is, h = √12 = about 3.5 cm.

This video tutorial will help users get an idea of ​​the Pyramid theme. Correct pyramid. In this lesson we will get acquainted with the concept of a pyramid and give it a definition. Let's consider what a regular pyramid is and what properties it has. Then we prove the theorem about the lateral surface of a regular pyramid.

In this lesson we will get acquainted with the concept of a pyramid and give it a definition.

Consider a polygon A 1 A 2...A n, which lies in the α plane, and the point P, which does not lie in the α plane (Fig. 1). Let's connect the dots P with peaks A 1, A 2, A 3, … A n. We get n triangles: A 1 A 2 R, A 2 A 3 R and so on.

Definition. Polyhedron RA 1 A 2 ...A n, made up of n-square A 1 A 2...A n And n triangles RA 1 A 2, RA 2 A 3 …RA n A n-1 is called n-coal pyramid. Rice. 1.

Rice. 1

Consider a quadrangular pyramid PABCD(Fig. 2).

R- the top of the pyramid.

ABCD- the base of the pyramid.

RA- side rib.

AB- base rib.

From point R let's drop the perpendicular RN to the base plane ABCD. The perpendicular drawn is the height of the pyramid.

Rice. 2

The full surface of the pyramid consists of the lateral surface, that is, the area of ​​​​all lateral faces, and the area of ​​the base:

S full = S side + S main

A pyramid is called correct if:

• its base is a regular polygon;
• the segment connecting the top of the pyramid with the center of the base is its height.

Explanation using the example of a regular quadrangular pyramid

Consider a regular quadrangular pyramid PABCD(Fig. 3).

R- the top of the pyramid. Base of the pyramid ABCD- a regular quadrilateral, that is, a square. Dot ABOUT, the point of intersection of the diagonals, is the center of the square. Means, RO is the height of the pyramid.

Rice. 3

Explanation: in the correct n In a triangle, the center of the inscribed circle and the center of the circumcircle coincide. This center is called the center of the polygon. Sometimes they say that the vertex is projected into the center.

The height of the lateral face of a regular pyramid drawn from its vertex is called apothem and is designated h a.

1. all lateral edges of a regular pyramid are equal;

2. The side faces are equal isosceles triangles.

We will give a proof of these properties using the example of a regular quadrangular pyramid.

Given: PABCD- regular quadrangular pyramid,

ABCD- square,

RO- height of the pyramid.

Prove:

1. RA = PB = RS = PD

2.∆ABP = ∆BCP =∆CDP =∆DAP See Fig. 4.

Rice. 4

Proof.

RO- height of the pyramid. That is, straight RO perpendicular to the plane ABC, and therefore direct JSC, VO, SO And DO lying in it. So triangles ROA, ROV, ROS, ROD- rectangular.

Consider a square ABCD. From the properties of a square it follows that AO = VO = CO = DO.

Then the right triangles ROA, ROV, ROS, ROD leg RO- general and legs JSC, VO, SO And DO are equal, which means that these triangles are equal on two sides. From the equality of triangles follows the equality of segments, RA = PB = RS = PD. Point 1 has been proven.

Segments AB And Sun are equal because they are sides of the same square, RA = PB = RS. So triangles AVR And VSR - isosceles and equal on three sides.

In a similar way we find that triangles ABP, VCP, CDP, DAP are isosceles and equal, as required to be proved in paragraph 2.

The area of ​​the lateral surface of a regular pyramid is equal to half the product of the perimeter of the base and the apothem:

To prove this, let’s choose a regular triangular pyramid.

Given: RAVS- regular triangular pyramid.

AB = BC = AC.

RO- height.

Prove: . See Fig. 5.

Rice. 5

Proof.

RAVS- regular triangular pyramid. That is AB= AC = BC. Let ABOUT- center of the triangle ABC, Then RO is the height of the pyramid. At the base of the pyramid lies an equilateral triangle ABC. Note that .

Triangles RAV, RVS, RSA- equal isosceles triangles (by property). A triangular pyramid has three side faces: RAV, RVS, RSA. This means that the area of ​​the lateral surface of the pyramid is:

S side = 3S RAW

The theorem has been proven.

The radius of a circle inscribed at the base of a regular quadrangular pyramid is 3 m, the height of the pyramid is 4 m. Find the area of ​​the lateral surface of the pyramid.

Given: regular quadrangular pyramid ABCD,

ABCD- square,

r= 3 m,

RO- height of the pyramid,

RO= 4 m.

Find: S side. See Fig. 6.

Rice. 6

Solution.

According to the proven theorem, .

Let's first find the side of the base AB. We know that the radius of a circle inscribed at the base of a regular quadrangular pyramid is 3 m.

Then, m.

Find the perimeter of the square ABCD with a side of 6 m:

Consider a triangle BCD. Let M- middle of the side DC. Because ABOUT- middle BD, That (m).

Triangle DPC- isosceles. M- middle DC. That is, RM- median, and therefore height in the triangle DPC. Then RM- apothem of the pyramid.

RO- height of the pyramid. Then, straight RO perpendicular to the plane ABC, and therefore direct OM, lying in it. Let's find the apothem RM from a right triangle ROM.

Now we can find the lateral surface of the pyramid:

Answer: 60 m2.

The radius of the circle circumscribed around the base of a regular triangular pyramid is equal to m. The area of ​​the lateral surface is 18 m 2. Find the length of the apothem.

Given: ABCP- regular triangular pyramid,

AB = BC = SA,

R= m,

S side = 18 m2.

Find: . See Fig. 7.

Rice. 7

Solution.

In a right triangle ABC The radius of the circumscribed circle is given. Let's find a side AB this triangle using the theorem of sines.

Knowing the side of a regular triangle (m), we find its perimeter.

By the theorem on the lateral surface area of ​​a regular pyramid, where h a- apothem of the pyramid. Then:

Answer: 4 m.

So, we looked at what a pyramid is, what a regular pyramid is, and we proved the theorem about the lateral surface of a regular pyramid. In the next lesson we will get acquainted with the truncated pyramid.

References

1. Geometry. Grades 10-11: textbook for students of general education institutions (basic and specialized levels) / I. M. Smirnova, V. A. Smirnov. - 5th ed., rev. and additional - M.: Mnemosyne, 2008. - 288 p.: ill.
2. Geometry. Grades 10-11: Textbook for general education institutions / Sharygin I. F. - M.: Bustard, 1999. - 208 pp.: ill.
3. Geometry. Grade 10: Textbook for general education institutions with in-depth and specialized study of mathematics /E. V. Potoskuev, L. I. Zvalich. - 6th ed., stereotype. - M.: Bustard, 008. - 233 p.: ill.

1. Internet portal "Yaklass" ()
2. Internet portal “Festival of pedagogical ideas “First of September” ()
3. Internet portal “Slideshare.net” ()

Homework

1. Can a regular polygon be the base of an irregular pyramid?
2. Prove that disjoint edges of a regular pyramid are perpendicular.
3. Find the value of the dihedral angle at the side of the base of a regular quadrangular pyramid if the apothem of the pyramid is equal to the side of its base.
4. RAVS- regular triangular pyramid. Construct the linear angle of the dihedral angle at the base of the pyramid.

Students encounter the concept of a pyramid long before studying geometry. The fault lies with the famous great Egyptian wonders of the world. Therefore, when starting to study this wonderful polyhedron, most students already clearly imagine it. All the above-mentioned attractions have the correct shape. What's happened regular pyramid, and what properties it has will be discussed further.

Definition

There are quite a lot of definitions of a pyramid. Since ancient times, it has been very popular.

For example, Euclid defined it as a bodily figure consisting of planes that, starting from one, converge at a certain point.

Heron provided a more precise formulation. He insisted that this was the figure that has a base and planes in the form of triangles, converging at one point.

Based on the modern interpretation, the pyramid is represented as a spatial polyhedron, consisting of a certain k-gon and k flat triangular figures, having one common point.

Let's look at it in more detail, what elements does it consist of:

• The k-gon is considered the basis of the figure;
• 3-gonal shapes protrude as the edges of the side part;
• the upper part from which the side elements originate is called the apex;
• all segments connecting a vertex are called edges;
• if a straight line is lowered from the vertex to the plane of the figure at an angle of 90 degrees, then its part contained in the internal space is the height of the pyramid;
• in any lateral element, a perpendicular, called an apothem, can be drawn to the side of our polyhedron.

The number of edges is calculated using the formula 2*k, where k is the number of sides of the k-gon. How many faces a polyhedron such as a pyramid has can be determined using the expression k+1.

Important! A pyramid of regular shape is a stereometric figure whose base plane is a k-gon with equal sides.

Basic properties

Correct pyramid has many properties, which are unique to her. Let's list them:

1. The basis is a figure of the correct shape.
2. The edges of the pyramid that limit the side elements have equal numerical values.
3. The side elements are isosceles triangles.
4. The base of the height of the figure falls at the center of the polygon, while it is simultaneously the central point of the inscribed and circumscribed.
5. All side ribs are inclined to the plane of the base at the same angle.
6. All side surfaces have the same angle of inclination relative to the base.

Thanks to all of the listed properties, performing element calculations is much simpler. Based on the above properties, we pay attention to two signs:

1. In the case when the polygon fits into a circle, the side faces will have equal angles with the base.
2. When describing a circle around a polygon, all edges of the pyramid emanating from the vertex will have equal lengths and equal angles with the base.

The basis is a square

Regular quadrangular pyramid - a polyhedron whose base is a square.

It has four side faces, which are isosceles in appearance.

A square is depicted on a plane, but is based on all the properties of a regular quadrilateral.

For example, if it is necessary to relate the side of a square with its diagonal, then use the following formula: the diagonal is equal to the product of the side of the square and the square root of two.

It is based on a regular triangle

A regular triangular pyramid is a polyhedron whose base is a regular 3-gon.

If the base is a regular triangle and the side edges are equal to the edges of the base, then such a figure called a tetrahedron.

All faces of a tetrahedron are equilateral 3-gons. In this case, you need to know some points and not waste time on them when calculating:

• the angle of inclination of the ribs to any base is 60 degrees;
• the size of all internal faces is also 60 degrees;
• any face can act as a base;
• , drawn inside the figure, these are equal elements.

Sections of a polyhedron

In any polyhedron there are several types of sections flat. Often in a school geometry course they work with two:

• axial;
• parallel to the basis.

An axial section is obtained by intersecting a polyhedron with a plane that passes through the vertex, side edges and axis. In this case, the axis is the height drawn from the vertex. The cutting plane is limited by the lines of intersection with all faces, resulting in a triangle.

Attention! In a regular pyramid, the axial section is an isosceles triangle.

If the cutting plane runs parallel to the base, then the result is the second option. In this case, we have a cross-sectional figure similar to the base.

For example, if the base is a square, then the section parallel to the base will also be a square, only of smaller dimensions.

When solving problems under this condition, they use signs and properties of similarity of figures, based on Thales' theorem. First of all, it is necessary to determine the similarity coefficient.

If the plane is drawn parallel to the base and it cuts off the upper part of the polyhedron, then a regular truncated pyramid is obtained in the lower part. Then the bases of a truncated polyhedron are said to be similar polygons. In this case, the side faces are isosceles trapezoids. The axial section is also isosceles.

In order to determine the height of a truncated polyhedron, it is necessary to draw the height in the axial section, that is, in the trapezoid.

Surface areas

The main geometric problems that have to be solved in a school geometry course are finding the surface area and volume of a pyramid.

There are two types of surface area values:

• area of ​​the side elements;
• area of ​​the entire surface.

From the name itself it is clear what we are talking about. The side surface includes only the side elements. It follows from this that to find it, you simply need to add up the areas of the lateral planes, that is, the areas of isosceles 3-gons. Let's try to derive the formula for the area of ​​the side elements:

1. The area of ​​an isosceles 3-gon is equal to Str=1/2(aL), where a is the side of the base, L is the apothem.
2. The number of lateral planes depends on the type of k-gon at the base. For example, a regular quadrangular pyramid has four lateral planes. Therefore, it is necessary to add the areas of four figures Sside=1/2(aL)+1/2(aL)+1/2(aL)+1/2(aL)=1/2*4a*L. The expression is simplified in this way because the value is 4a = Rosn, where Rosn is the perimeter of the base. And the expression 1/2*Rosn is its semi-perimeter.
3. So, we conclude that the area of ​​the lateral elements of a regular pyramid is equal to the product of the semi-perimeter of the base and the apothem: Sside = Rosn * L.

The area of ​​the total surface of the pyramid consists of the sum of the areas of the side planes and the base: Sp.p. = Sside + Sbas.

As for the area of ​​the base, here the formula is used according to the type of polygon.

Volume of a regular pyramid equal to the product of the area of ​​the base plane and the height divided by three: V=1/3*Sbas*H, where H is the height of the polyhedron.

What is a regular pyramid in geometry

Properties of a regular quadrangular pyramid

The text of the work is posted without images and formulas.
The full version of the work is available in the "Work Files" tab in PDF format

Introduction

When we encounter the word “pyramid,” our associative memory takes us to Egypt. If we talk about early architectural monuments, we can say that their number is at least several hundred. An Arab writer of the 13th century said: “Everything in the world is afraid of time, and time is afraid of the pyramids.” The pyramids are the only one of the seven wonders of the world that has survived to our time, before the era of computer technology. However, researchers have still not been able to find the keys to all their mysteries. The more we learn about the pyramids, the more questions we have. Pyramids are of interest to historians, physicists, biologists, physicians, philosophers, etc. They arouse great interest and encourage a deeper study of their properties, both from mathematical and other points of view (historical, geographical, etc.).

That's why purpose Our research was to study the properties of the pyramid from different points of view. We have identified as intermediate goals: consideration of the properties of the pyramid from the point of view of mathematics, study of hypotheses about the existence of secrets and mysteries of the pyramid, as well as the possibilities of its application.

Object The study in this work is a pyramid.

Item research: features and properties of the pyramid.

Tasks research:

Study popular scientific literature on the topic of research.

Consider the pyramid as a geometric body.

Determine the properties and features of the pyramid.

Find material confirming the application of the properties of the pyramid in various fields of science and technology.

Methods research: analysis, synthesis, analogy, mental modeling.

Expected result of the work there should be structured information about the pyramid, its properties and application possibilities.

Stages of project preparation:

Determining the project topic, goals and objectives.

Studying and collecting material.

Drawing up a project plan.

Formulation of the expected result of activity on the project, including the assimilation of new material, the formation of knowledge, skills and abilities in the subject activity.

Presentation of research results.

Reflection

Pyramid as a geometric body

Let's consider the origins of the word and term “ pyramid" It is immediately worth noting that the “pyramid” or “ pyramid"(English), " pyramid"(French, Spanish and Slavic languages), "pyramide"(German) is a Western term with its origins in ancient Greece. In ancient Greek πύραμίς (“p iramis"and many more. h. Πύραμίδες « pyramides") has several meanings. The ancient Greeks called pyramid» wheat cake that resembled the shape of Egyptian buildings. Later the word came to mean “a monumental structure with a square area at the base and sloping sides meeting at the top. The etymological dictionary indicates that the Greek "pyramis" comes from the Egyptian " pimar." First written interpretation of the word "pyramid" found in Europe in 1555 and means: “one of the types of ancient structures of kings.” After the discovery of the pyramids in Mexico and with the development of science in the 18th century, the pyramid became not just an ancient architectural monument, but also a regular geometric figure with four symmetrical sides (1716). Pyramid geometry began in Ancient Egypt and Babylon, but was actively developed in Ancient Greece. The first to establish the volume of the pyramid was Democritus, and it was proved by Eudoxus of Cnidus.

The first definition belongs to the ancient Greek mathematician, the author of theoretical treatises on mathematics that have come down to us, Euclid. In the XII volume of his "Principles" he defines a pyramid as a solid figure bounded by planes that from one plane (base) converge at one point (apex). But this definition was criticized already in ancient times. So Heron proposed the following definition of a pyramid: “It is a figure bounded by triangles converging at one point and the base of which is a polygon.”

There is a definition by the French mathematician Adrien Marie Legendre, who in 1794 in his work “Elements of Geometry” defines a pyramid as follows: “A pyramid is a solid figure formed by triangles converging at one point and ending on different sides of a flat base.”

Modern dictionaries interpret the term “pyramid” as follows:

Analyzing the definitions of the pyramid, we can conclude that all sources have similar formulations:

A pyramid is a polyhedron whose base is a polygon and the remaining faces are triangles that have a common vertex. Based on the number of base angles, pyramids are classified as triangular, quadrangular, etc.

Polygon A 1 A 2 A 3 ... An is the base of the pyramid, and triangles RA 1 A 2 , RA 2 A 3 , ..., RANA 1 are the side faces of the pyramid, P is the top of the pyramid, segments RA 1 , RA 2 , ..., RAN - lateral ribs.

The perpendicular drawn from the top of the pyramid to the plane of the base is called heighth pyramids.

In addition to an arbitrary pyramid, there is a regular pyramid, at the base of which is a regular polygon and a truncated pyramid.

Area The total surface of a pyramid is the sum of the areas of all its faces. Sfull = S side + S main, where S side is the sum of the areas of the side faces.

Volume pyramid is found by the formula: V=1/3S main.h, where S main. - base area, h - height.

TO properties of the pyramid include:

When all the side edges are of the same size, then it is easy to describe a circle around the base of the pyramid, with the top of the pyramid projected into the center of this circle; the side ribs form equal angles with the plane of the base; Moreover, the opposite is also true, i.e. when the side ribs form equal angles with the plane of the base, or when a circle can be described around the base of the pyramid and the top of the pyramid will be projected into the center of this circle, it means that all the side edges of the pyramid are the same size.

When the side faces have an angle of inclination to the plane of the base of the same magnitude, then it is easy to describe a circle around the base of the pyramid, and the top of the pyramid will be projected into the center of this circle; the heights of the side faces are of equal length; the area of ​​the side surface is equal to half the product of the perimeter of the base and the height of the side face.

The pyramid is called correct, if its base is a regular polygon, and its vertex is projected to the center of the base. The lateral faces of a regular pyramid are equal, isosceles triangles (Fig. 2a). Axis of a regular pyramid is the straight line containing its height. Apothem - the height of the side face of a regular pyramid drawn from its vertex.

Square side face of a regular pyramid is expressed as follows: Sside. =1/2P h, where P is the perimeter of the base, h is the height of the side face (apothem of a regular pyramid). If the pyramid is intersected by the plane A’B’C’D’, parallel to the base, then the lateral edges and height are divided by this plane into proportional parts; in cross-section, a polygon A’B’C’D’ is obtained, similar to the base; The cross-sectional areas and bases are related as the squares of their distances from the vertex.

Truncated pyramid is obtained by cutting off its upper part from the pyramid with a plane parallel to the base (Fig. 2b). The bases of the truncated pyramid are similar polygons ABCD and A`B`C`D`, the side faces are trapezoids. The height of a truncated pyramid is the distance between the bases. The volume of a truncated pyramid is found by the formula: V = 1/3 h (S + + S’), where S and S’ are the areas of the bases ABCD and A’B’C’D’, h is the height.

The bases of a regular truncated n-gonal pyramid are regular n-gons. The lateral surface area of ​​a regular truncated pyramid is expressed as follows: Sside. = ½(P+P’)h, where P and P’ are the perimeters of the bases, h is the height of the side face (apothem of a regular truncated pyramid)

Sections of a pyramid by planes passing through its apex are triangles. The section passing through two non-adjacent lateral edges of the pyramid is called a diagonal section. If the section passes through a point on the side edge and the side of the base, then its trace to the plane of the base of the pyramid will be this side. A section passing through a point lying on the face of the pyramid and a given section trace on the base plane, then the construction should be carried out as follows: find the point of intersection of the plane of the given face and the section trace of the pyramid and designate it; construct a straight line passing through a given point and the resulting intersection point; repeat these steps for the next faces.

Rectangular pyramid - This is a pyramid in which one of the side edges is perpendicular to the base. In this case, this edge will be the height of the pyramid (Fig. 2c).

Regular triangular pyramid is a pyramid whose base is a regular triangle, and the apex is projected into the center of the base. A special case of a regular triangular pyramid is tetrahedron. (Fig. 2a)

Let's consider theorems connecting the pyramid with other geometric bodies.

Sphere

A sphere can be described around a pyramid when at the base of the pyramid there is a polygon around which a circle can be described (a necessary and sufficient condition). The center of the sphere will be the intersection point of the planes passing through the midpoints of the edges of the pyramid perpendicular to them. From this theorem it follows that a sphere can be described both around any triangular and around any regular pyramid; A sphere can be inscribed into a pyramid when the bisector planes of the internal dihedral angles of the pyramid intersect at one point (a necessary and sufficient condition). This point will be the center of the sphere.

Cone

A cone is said to be inscribed in a pyramid if their vertices coincide and its base is inscribed in the base of the pyramid. Moreover, it is possible to fit a cone into a pyramid only when the apothems of the pyramid are equal to each other (a necessary and sufficient condition); A cone is said to be described near a pyramid when their vertices coincide and its base is described near the base of the pyramid. Moreover, it is possible to describe a cone near a pyramid only when all the lateral edges of the pyramid are equal to each other (a necessary and sufficient condition); The heights of such cones and pyramids are equal to each other.

Cylinder

A cylinder is said to be inscribed in a pyramid if one of its bases coincides with a circle inscribed in the section of the pyramid by a plane parallel to the base, and the other base belongs to the base of the pyramid. A cylinder is said to be described near a pyramid if the vertex of the pyramid belongs to one of its bases, and its other base is described near the base of the pyramid. Moreover, it is possible to describe a cylinder near a pyramid only if there is an inscribed polygon at the base of the pyramid (a necessary and sufficient condition).

Very often in their research scientists use the properties of the pyramid with Golden Ratio proportions. We will look at how the golden ratio ratios were used when building pyramids in the next paragraph, and here we will dwell on the definition of the golden ratio.

The mathematical encyclopedic dictionary gives the following definition Golden ratio- this is the division of segment AB into two parts in such a way that its larger part AC is the average proportional between the entire segment AB and its smaller part CD.

Algebraic determination of the Golden section of the segment AB = a is reduced to solving the equation a:x = x:(a-x), from which x is approximately equal to 0.62a. The ratio x can be expressed as fractions n/n+1= 0,618, where n is the Fibonacci number numbered n.

The golden ratio is often used in works of art, architecture, and is found in nature. Vivid examples are the sculpture of Apollo Belvedere and the Parthenon. During the construction of the Parthenon, the ratio of the height of the building to its length was used and this ratio is 0.618. Objects around us also provide examples of the Golden Ratio, for example, the bindings of many books also have a width-to-length ratio close to 0.618.

Thus, having studied popular scientific literature on the research problem, we came to the conclusion that a pyramid is a polyhedron, the base of which is a polygon, and the remaining faces are triangles with a common vertex. We examined the elements and properties of the pyramid, its types and relationship with the proportions of the Golden Ratio.

2. Features of the pyramid

So in the Big Encyclopedic Dictionary it is written that a pyramid is a monumental structure that has the geometric shape of a pyramid (sometimes stepped or tower-shaped). Pyramids were the name given to the tombs of ancient Egyptian pharaohs of the 3rd - 2nd millennium BC. e., as well as pedestals of temples in Central and South America associated with cosmological cults. Among the grandiose pyramids of Egypt, the Great Pyramid of Pharaoh Cheops occupies a special place. Before we begin to analyze the shape and size of the Cheops pyramid, we should remember what system of measures the Egyptians used. The Egyptians had three units of length: a “cubit” (466 mm), which was equal to seven “palms” (66.5 mm), which in turn was equal to four “fingers” (16.6 mm).

Most researchers agree that the length of the side of the base of the pyramid, for example, GF, is equal to L = 233.16 m. This value corresponds almost exactly to 500 “cubits”. Full compliance with 500 “elbows” will occur if the length of the “elbow” is considered equal to 0.4663 m.

The height of the pyramid (H) is estimated by researchers variously from 146.6 to 148.2 m. And depending on the accepted height of the pyramid, all the relationships of its geometric elements change. What is the reason for the differences in estimates of the height of the pyramid? The fact is that the Cheops pyramid is truncated. Its upper platform today measures approximately 10x10 m, but a century ago it was 6x6 m. Obviously, the top of the pyramid was dismantled, and it does not correspond to the original one. When assessing the height of the pyramid, it is necessary to take into account such a physical factor as the settlement of the structure. Over a long period of time, under the influence of colossal pressure (reaching 500 tons per 1 m 2 of the lower surface), the height of the pyramid decreased compared to its original height. The original height of the pyramid can be recreated by finding a basic geometric idea.

In 1837, the English Colonel G. Wise measured the angle of inclination of the faces of the pyramid: it turned out to be equal to a = 51°51". This value is still recognized by most researchers today. The indicated value of the angle corresponds to a tangent (tg a) equal to 1.27306. This value corresponds to the ratio of the height of the pyramid AC to half of its base CB, that is, AC / CB = H / (L / 2) = 2H / L.

And here the researchers were in for a big surprise! The fact is that if we take the square root of the golden ratio, we get the following result = 1.272. Comparing this value with the value tg a = 1.27306, we see that these values ​​are very close to each other. If we take the angle a = 51°50", that is, reduce it by only one arc minute, then the value of a will become equal to 1.272, that is, it will coincide with the value. It should be noted that in 1840 G. Wise repeated his measurements and clarified , that the value of angle a = 51°50".

These measurements led the researchers to the following interesting hypothesis: the triangle ACB of the Cheops pyramid was based on the ratio AC / CB = 1.272.

Let us now consider a right triangle ABC, in which the ratio of the legs AC / CB = . If we now denote the lengths of the sides of rectangle ABC by x, y, z, and also take into account that the ratio y/x =, then in accordance with the Pythagorean theorem, the length z can be calculated using the formula:

If we accept x = 1, y = , then:

A right triangle in which the sides are in the ratio t::1 is called a “golden” right triangle.

Then, if we take as a basis the hypothesis that the main “geometric idea” of the Cheops pyramid is a “golden” right triangle, then from here we can easily calculate the “design” height of the Cheops pyramid. It is equal to:

H = (L/2)/= 148.28 m.

Let us now derive some other relations for the Cheops pyramid, arising from the “golden” hypothesis. In particular, we will find the ratio of the outer area of ​​the pyramid to the area of ​​its base. To do this, we take the length of the leg CB as one, that is: CB = 1. But then the length of the side of the base of the pyramid is GF = 2, and the area of ​​the base EFGH will be equal to S EFGH = 4.

Let us now calculate the area of ​​the side face of the Cheops pyramid S D . Since the height AB of triangle AEF is equal to t, the area of ​​the side face will be equal to S D = t. Then the total area of ​​all four lateral faces of the pyramid will be equal to 4t, and the ratio of the total outer area of ​​the pyramid to the area of ​​the base will be equal to the golden ratio. This is the main geometric mystery of the Cheops pyramid.

And also, during the construction of the Egyptian pyramids, it was found that a square built at the height of the pyramid is exactly equal to the area of ​​​​each of the side triangles. This is confirmed by the latest measurements.

We know that the relationship between the length of a circle and its diameter is a constant value, well known to modern mathematicians and schoolchildren - this is the number “Pi” = 3.1416... But if we add up the four sides of the base of the Cheops pyramid, we get 931.22 m. Dividing this number by twice the height of the pyramid (2x148.208), we get 3.1416..., that is, the number “Pi”. Consequently, the Cheops pyramid is a one-of-a-kind monument that represents the material embodiment of the number “Pi,” which plays an important role in mathematics.

Thus, the presence of the golden ratio in the dimensions of the pyramid - the ratio of the double side of the pyramid to its height - is a number very close in value to the number π. This is undoubtedly also a feature. Although many authors believe that this coincidence is accidental, since the fraction 14/11 is “a good approximation for both the square root of the golden ratio and the ratio of the areas of a square and the circle inscribed in it.”

However, it is incorrect to talk here only about the Egyptian pyramids. There are not only Egyptian pyramids, there is a whole network of pyramids on Earth. The main monuments (Egyptian and Mexican pyramids, Easter Island and the Stonehenge complex in England) at first glance are scattered randomly across our planet. But if the Tibetan complex of pyramids is included in the study, then a strict mathematical system of their location on the surface of the Earth appears. Against the background of the Himalayan range, a pyramidal formation clearly stands out - Mount Kailash. The location of the city of Kailash, the Egyptian and Mexican pyramids is very interesting, namely - if you connect the city of Kailash with the Mexican pyramids, then the line connecting them goes to Easter Island. If you connect the city of Kailash with the Egyptian pyramids, then the line of their connection again goes to Easter Island. Exactly one-fourth of the globe was outlined. If we connect the Mexican and Egyptian pyramids, we will see two equal triangles. If you find their areas, then their sum is equal to one-fourth the area of ​​the globe.

An indisputable connection between the Tibetan pyramid complex has been revealed with other structures antiquity - Egyptian and Mexican pyramids, the colossi of Easter Island and the Stonehenge complex in England. The height of the main pyramid of Tibet - Mount Kailash - is 6714 meters. The distance from Kailash to the North Pole is 6714 kilometers, the distance from Kailash to Stonehenge is 6714 kilometers If we put these on the globe from the North Pole 6714 kilometers, then we will get to the so-called Devil's Tower, which looks like a truncated pyramid. And finally, exactly 6714 kilometers from Stonehenge to the Bermuda Triangle.

As a result of these studies, we can conclude that there is a pyramidal-geographical system on Earth.

Thus, the features include the ratio of the total outer area of ​​the pyramid to the area of ​​the base will be equal to the golden ratio; the presence in the dimensions of the pyramid of the golden ratio - the ratio of the double side of the pyramid to its height - is a number very close in value to the number π, i.e. the Cheops pyramid is a one-of-a-kind monument that represents the material embodiment of the number “Pi”; the existence of a pyramidal-geographical system.

3. Other properties and uses of the pyramid.

Let's consider the practical application of this geometric figure. For example, hologram. First, let's look at what holography is. Holography - a set of technologies for accurately recording, reproducing and reshaping the wave fields of optical electromagnetic radiation, a special photographic method in which, using a laser, images of three-dimensional objects are recorded and then reconstructed, highly similar to real ones. A hologram is a product of holography, a three-dimensional image created using a laser that reproduces an image of a three-dimensional object. Using a regular truncated tetrahedral pyramid, you can recreate an image - a hologram. A photo file and a regular truncated tetrahedral pyramid from a translucent material are created. A small indentation is made from the bottommost pixel and the middle one relative to the ordinate axis. This point will be the middle of the side of the square formed by the section. The photograph is multiplied, and its copies are positioned in the same way relative to the other three sides. Place the pyramid on the square with its cross section down so that it coincides with the square. The monitor generates a light wave, each of four identical photographs, being in a plane that is a projection of the face of the pyramid, falls on the face itself. As a result, on each of the four faces we have identical images, and since the material from which the pyramid is made has the property of transparency, the waves seem to be refracted, meeting in the center. As a result, we get the same interference pattern of a standing wave, the central axis, or the axis of rotation of which is the height of a regular truncated pyramid. This method also works with video images, since the principle of operation remains unchanged.

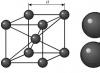
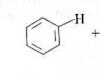
Considering special cases, you can see that the pyramid is widely used in everyday life, even in the household. The pyramidal shape is found frequently, primarily in nature: plants, crystals, the methane molecule has the shape of a regular triangular pyramid - a tetrahedron, The unit cell of a diamond crystal is also a tetrahedron, with carbon atoms located in the center and four vertices. Pyramids are found at home and in children's toys. Buttons and computer keyboards are often like a quadrangular truncated pyramid. They can be seen in the form of elements of buildings or architectural structures themselves, like translucent roof structures.

Let's look at some more examples of the use of the term "pyramid"

Ecological pyramids- these are graphic models (usually in the form of triangles) reflecting the number of individuals (pyramid of numbers), the amount of their biomass (pyramid of biomass) or the energy contained in them (pyramid of energy) at each trophic level and indicating a decrease in all indicators with increasing trophic level level

Information pyramid. It reflects the hierarchy of different types of information. The provision of information is structured according to the following pyramidal scheme: at the top are the main indicators by which you can clearly track the pace of the enterprise’s movement towards the chosen goal. If something is wrong, then you can go to the middle level of the pyramid - generalized data. They clarify the picture for each indicator individually or in conjunction with each other. Using this data, you can determine the possible location of a failure or problem. For more complete information, you need to turn to the base of the pyramid - a detailed description of the state of all processes in numerical form. This data helps identify the cause of the problem so that it can be corrected and avoided in the future.

Bloom's Taxonomy. Bloom's taxonomy offers a classification of tasks in the form of a pyramid that teachers set for students, and, accordingly, learning goals. She divides educational goals into three areas: cognitive, affective and psychomotor. Within each individual sphere, in order to move to a higher level, experience of the previous levels distinguished in this sphere is necessary.

Financial pyramid- a specific phenomenon of economic development. The name “pyramid” clearly illustrates the situation when people “at the bottom” of the pyramid give money to the small top. Moreover, each new participant pays to increase the possibility of his promotion to the top of the pyramid

Pyramid of needs Maslow reflects one of the most popular and well-known theories of motivation - the theory of hierarchy needs. Maslow distributed needs as they increase, explaining this construction by the fact that a person cannot experience high-level needs while he needs more primitive things. As lower-lying needs are satisfied, higher-level needs become more and more relevant, but this does not mean that the place of the previous need is taken by a new one only when the previous one is fully satisfied.

Another example of the use of the term “pyramid” is food pyramid - a schematic representation of the principles of healthy eating developed by nutritionists. Foods at the base of the pyramid should be eaten as often as possible, while foods at the top of the pyramid should be avoided or consumed in limited quantities.

Thus, all of the above shows the variety of uses of the pyramid in our lives. Perhaps the pyramid has a much higher purpose, and is intended for something greater than the practical uses that are now discovered.

Conclusion

We constantly encounter pyramids in our lives - these are the ancient Egyptian pyramids and toys that children play with; objects of architecture and design, natural crystals; viruses that can only be seen with an electron microscope. Over the many millennia of their existence, the pyramids have become a kind of symbol, personifying man’s desire to reach the pinnacle of knowledge.

During the study, we determined that pyramids are a fairly common phenomenon throughout the globe.

We studied popular scientific literature on the topic of research, examined various interpretations of the term “pyramid”, determined that in a geometric sense, a pyramid is a polyhedron, the base of which is a polygon, and the remaining faces are triangles that have a common vertex. We studied the types of pyramids (regular, truncated, rectangular), elements (apothem, side faces, side edges, apex, height, base, diagonal section) and the properties of geometric pyramids when the side edges are equal and when the side faces are inclined to the plane of the base at the same angle. We examined theorems connecting the pyramid with other geometric bodies (sphere, cone, cylinder).

We included the following features of the pyramid:

the ratio of the total outer area of ​​the pyramid to the area of ​​the base will be equal to the golden ratio;

the presence in the dimensions of the pyramid of the golden ratio - the ratio of the double side of the pyramid to its height - is a number very close in value to the number π, i.e. the Cheops pyramid is a one-of-a-kind monument that represents the material embodiment of the number “Pi”;

the existence of a pyramidal-geographical system.

We studied the modern use of this geometric figure. We looked at how the pyramid and the hologram are connected, and noticed that the pyramidal shape is most often found in nature (plants, crystals, methane molecules, the structure of the diamond lattice, etc.). Throughout the study, we encountered material confirming the use of the properties of the pyramid in various fields of science and technology, in people’s everyday lives, in the analysis of information, in economics and in many other areas. And they came to the conclusion that perhaps the pyramids have a much higher purpose, and are intended for something greater than the practical ways of using them that are now discovered.

References.

Van der Waerden, Bartel Leendert. Awakening Science. Mathematics of Ancient Egypt, Babylon and Greece. [Text]/ B. L. Van der Waerden - KomKniga, 2007

Voloshinov A.V. Mathematics and art. [Text]/ A.V. Voloshinov - Moscow: “Enlightenment” 2000.

World History (encyclopedia for children). [Text]/ - M.: “Avanta+”, 1993.

Halogram . [Electronic resource] - https://hi-news.ru/tag/hologramma - article on the Internet

Geometry [Text]: Textbook. 10 - 11 grades for educational institutions Atanasyan L.S., V.F. Butuzov and others - 22nd edition. - M.: Education, 2013.

Coppens F. New era of pyramids. [Text]/ F. Coppens - Smolensk: Rusich, 2010

Mathematical encyclopedic dictionary. [Text]/ A. M. Prokhorov et al. - M.: Soviet Encyclopedia, 1988.

Muldashev E. R. The world system of pyramids and monuments of antiquity saved us from the end of the world, but ... [Text] / E. R. Muldashev - M.: “AiF-Print”; M.: “OLMA-PRESS”; St. Petersburg: Publishing House "Neva"; 2003.

Perelman Ya. I. Entertaining arithmetic. [Text]/ Ya. I. Perelman - M.: Tsentrpoligraf, 2017

Reichard G. Pyramids. [Text]/ Hans Reichard - M.: Slovo, 1978

Terra-Lexicon. Illustrated encyclopedic dictionary. [Text]/ - M.: TERRA, 1998.

Tompkins P. Secrets of the Great Pyramid of Cheops. [Text]/ Peter Tompkins. - M.: “Centropolygraph”, 2008

Uvarov V. Magic properties of pyramids. [Text]/ V. Uvarov - Lenizdat, 2006.

Sharygin I.F.. Geometry grades 10-11. [Text]/ I.F. Sharygin:. - M: “Enlightenment”, 2000

Yakovenko M. The key to understanding the pyramid. [Electronic resource] - http://world-pyramids.com/russia/pyramid.html - article on the Internet