Using an online calculator you can find inflection points and convexity intervals of the function graph with the design of the solution in Word. Whether a function of two variables f(x1,x2) is convex is decided using the Hessian matrix.
Rules for entering functions:
The direction of convexity of the graph of a function. Inflection points
Definition: The curve y=f(x) is called convex downward in the interval (a; b) if it lies above the tangent at any point in this interval.Definition: The curve y=f(x) is said to be convex upward in the interval (a; b) if it lies below the tangent at any point in this interval. 
Definition: The intervals in which the graph of a function is convex up or down are called intervals of convexity of the graph of the function.
The convexity downward or upward of a curve that is a graph of the function y=f(x) is characterized by the sign of its second derivative: if in a certain interval f’’(x) > 0, then the curve is convex downward on this interval; if f’’(x)< 0, то кривая выпукла вверх на этом промежутке.
Definition: A point on the graph of a function y=f(x) separating the convexity intervals opposite directions of this graph is called the inflection point. 
Inflection points can only serve critical points II kind, i.e. points belonging to the domain of definition of the function y = f(x) at which the second derivative f’’(x) vanishes or has a discontinuity.
The rule for finding inflection points in the graph of a function y = f(x)
- Find the second derivative f’’(x) .
- Find critical points of the second kind of the function y=f(x), i.e. the point at which f''(x) vanishes or experiences a discontinuity.
- Investigate the sign of the second derivative f’’(x) in the interval into which the found critical points divide the domain of definition of the function f(x). If the critical point x 0 separates the convexity intervals of opposite directions, then x 0 is the abscissa of the inflection point of the function graph.
- Calculate the function values at the inflection points.
Example 1. Find the convexity intervals and inflection points of the following curve: f(x) = 6x 2 –x 3.
Solution: Find f ‘(x) = 12x – 3x 2 , f ‘’(x) = 12 – 6x.
Let's find the critical points of the second derivative by solving the equation 12-6x=0. x=2 . 
f(2) = 6*2 2 – 2 3 = 16
Answer: The function is convex upward for x∈(2; +∞) ; the function is convex downwards at x∈(-∞; 2) ; inflection point (2;16) .
Example 2. Does the function have inflection points: f(x)=x 3 -6x 2 +2x-1
Example 3. Find the intervals where the graph of the function is convex and curved: f(x)=x 3 -6x 2 +12x+4
At researching a function and plotting its graph at one stage we determine the inflection points and convexity intervals. This data along with intervals of increasing and decreasing allow you to schematically represent the graph of the function under study.
The further presentation assumes that you can do up to some order and different types.
Let's start studying the material with necessary definitions and concepts. Next, we will voice the connection between the value of the second derivative of a function on a certain interval and the direction of its convexity. After this, we will move on to the conditions that allow us to determine the inflection points of the function graph. According to the text we will give typical examples with detailed solutions.
Page navigation.
Convexity, concavity of a function, inflection point.
Definition.
convex down on the interval X if its graph is located not lower than the tangent to it at any point of the interval X.
Definition.
The function to be differentiated is called convex up on the interval X if its graph is located no higher than the tangent to it at any point in the interval X.
An upward convex function is often called convex, and convex down – concave.
Look at the drawing illustrating these definitions.
Definition.
The point is called inflection point of the function graph y=f(x) if at a given point there is a tangent to the graph of the function (it can be parallel to the Oy axis) and there is a neighborhood of the point within which to the left and right of the point M the graph of the function has different directions of convexity.
In other words, point M is called an inflection point of the graph of a function if there is a tangent at this point and the graph of the function changes the direction of the convexity, passing through it.
If necessary, refer to the section to recall the conditions for the existence of a non-vertical and vertical tangent.
The figure below shows some examples of inflection points (marked with red dots). Note that some functions may have no inflection points, while others may have one, several, or infinitely many inflection points.
Finding intervals of convexity of a function.
Let us formulate a theorem that allows us to determine the convexity intervals of a function.
Theorem.
If the function y=f(x) has a finite second derivative on the interval X and if the inequality holds 
(), then the graph of the function has a convexity directed downwards (upwards) by X.
This theorem allows you to find the intervals of concavity and convexity of a function; you only need to solve the inequalities and, respectively, on the domain of definition of the original function.
It should be noted that points at which the function y=f(x) is defined and the second derivative does not exist will be included in the concavity and convexity intervals.
Let's understand this with an example.
Example.
Find out the intervals on which the graph of the function 
has a convexity directed upward and a convexity directed downward.
Solution.
Function Domain- it's all a lot real numbers.
Let's find the second derivative. 
The domain of definition of the second derivative coincides with the domain of definition of the original function, therefore, to find out the intervals of concavity and convexity, it is enough to solve and accordingly. 
Consequently, the function is convex downwards on the interval and convex upwards on the interval .
Graphic illustration.
The part of the function graph in the convex interval is depicted in blue, in the concavity interval – in red.
Now let's consider an example when the domain of definition of the second derivative does not coincide with the domain of definition of the function. In this case, as we have already noted, points of the domain of definition at which a finite second derivative does not exist should be included in the intervals of convexity and (or) concavity.
Example.
Find the intervals of convexity and concavity of the graph of the function.
Solution.
Let's start with the domain of the function:
Let's find the second derivative: 
The domain of definition of the second derivative is the set 
. As you can see, x=0 belongs to the domain of the original function, but does not belong to the domain of the second derivative. Do not forget about this point; it will need to be included in the interval of convexity and (or) concavity.
Now we solve inequalities on the domain of definition of the original function. Let's apply. Numerator of expression 
goes to zero at 
or 
, denominator – at x = 0 or x = 1. We schematically plot these points on the number line and find out the sign of the expression on each of the intervals included in the domain of definition of the original function (it is shown as a shaded area on the lower number line). For a positive value we put a plus sign, for a negative value we put a minus sign. 
Thus,
And 
Therefore, by including the point x=0, we get the answer.
At 
the graph of the function has a convexity directed downward, with 
- convexity directed upward.
Graphic illustration.
The part of the graph of the function on the convexity interval is depicted in blue, on the concavity intervals - in red, the black dotted line is vertical asymptote.
Necessary and sufficient conditions for inflection.
Necessary condition for inflection.
Let's formulate necessary condition for inflection function graphics.
Let the graph of the function y=f(x) have an inflection at a point and have a continuous second derivative, then the equality holds.
From this condition it follows that the abscissa of the inflection points should be sought among those at which the second derivative of the function vanishes. BUT, this condition is not sufficient, that is, not all values in which the second derivative is equal to zero are abscissas of inflection points.
It should also be noted that the definition of an inflection point requires the existence of a tangent line, or a vertical one. What does this mean? And this means the following: the abscissas of the inflection points can be everything from the domain of definition of the function for which 
And 
. These are usually the points at which the denominator of the first derivative vanishes.
The first sufficient condition for inflection.
After all have been found that can be abscissas of inflection points, you should use the first sufficient condition for inflection function graphics.
Let the function y=f(x) be continuous at the point, have a tangent (possibly vertical) at it, and let this function have a second derivative in some neighborhood of the point. Then, if within this neighborhood to the left and right of , the second derivative has different signs, then is the inflection point of the function graph.
As you can see, the first sufficient condition does not require the existence of the second derivative at the point itself, but requires its existence in the neighborhood of the point.
Now let’s summarize all the information in the form of an algorithm.
Algorithm for finding inflection points of a function.
We find all the abscissas of possible inflection points of the function graph (or And ) and find out by passing through which the second derivative changes sign. Such values will be the abscissa of the inflection points, and the corresponding points will be the inflection points of the function graph.
Let's look at two examples of finding inflection points for clarification.
Example.
Find inflection points and intervals of convexity and concavity of the graph of a function 
.
Solution.
The domain of a function is the entire set of real numbers.
Let's find the first derivative: 
The domain of definition of the first derivative is also the entire set of real numbers, therefore the equalities And is not fulfilled for any .
Let's find the second derivative:
Let’s find out at what values of the argument x the second derivative goes to zero: 
Thus, the abscissas of possible inflection points are x=-2 and x=3.
Now it remains to check, using a sufficient sign of inflection, at which of these points the second derivative changes sign. To do this, plot the points x=-2 and x=3 on number axis and, as in generalized interval method, we place the signs of the second derivative over each interval. Under each interval, the direction of convexity of the function graph is shown schematically with arcs. 
The second derivative changes sign from plus to minus, passing through the point x=-2 from left to right, and changes sign from minus to plus, passing through x=3. Therefore, both x=-2 and x=3 are abscissas of the inflection points of the function graph. They correspond to the graph points and .
Taking another look at the number line and the signs of the second derivative on its intervals, we can draw conclusions about the intervals of convexity and concavity. The graph of a function is convex on the interval and concave on the intervals and .
Graphic illustration.
The part of the function graph on the convex interval is shown in blue, on the concavity interval – in red, and inflection points are shown as black dots.
Example.
Find the abscissa of all inflection points of the function graph 
.
Solution.
The domain of definition of this function is the entire set of real numbers.
Let's find the derivative. 
The first derivative, unlike the original function, is not defined at x=3. But 
And 
. Therefore, at the point with abscissa x=3 there is a vertical tangent to the graph of the original function. Thus, x=3 can be the abscissa of the inflection point of the function graph.
We find the second derivative, its domain of definition and the points at which it vanishes: 
We obtained two more possible abscissas of inflection points. We mark all three points on the number line and determine the sign of the second derivative on each of the resulting intervals. 
The second derivative changes sign when passing through each of the points, therefore, they are all abscissas of inflection points.
Graph of a function y=f(x) called convex on the interval (a; b), if it is located below any of its tangents on this interval.
Graph of a function y=f(x) called concave on the interval (a; b), if it is located above any of its tangents on this interval.
The figure shows a curve that is convex at (a; b) and concave on (b;c).
Examples.
Let us consider a sufficient criterion that allows us to determine whether the graph of a function in a given interval will be convex or concave.
Theorem. Let y=f(x) differentiable by (a; b). If at all points of the interval (a; b) second derivative of the function y = f(x) negative, i.e. f ""(x) < 0, то график функции на этом интервале выпуклый, если же f""(x) > 0 – concave.
Proof. Let us assume for definiteness that f""(x) < 0 и докажем, что график функции будет выпуклым.
Let's take the functions on the graph y = f(x) arbitrary point M0 with abscissa x 0 Î ( a; b) and draw through the point M0 tangent. Her equation. We must show that the graph of the function on (a; b) lies below this tangent, i.e. at the same value x ordinate of curve y = f(x) will be less than the ordinate of the tangent.
So, the equation of the curve is y = f(x). Let us denote the ordinate of the tangent corresponding to the abscissa x. Then . Consequently, the difference between the ordinates of the curve and the tangent for the same value x will .
Difference f(x) – f(x 0) transform according to Lagrange's theorem, where c between x And x 0.
Thus,
We again apply Lagrange’s theorem to the expression in square brackets: , where c 1 between c 0 And x 0. According to the conditions of the theorem f ""(x) < 0. Определим знак произведения второго и третьего сомножителей.
Thus, any point on the curve lies below the tangent to the curve for all values x And x 0 Î ( a; b), which means that the curve is convex. The second part of the theorem is proved in a similar way.
Examples.
Graph point continuous function, separating its convex part from the concave part, is called inflection point.
Obviously, at the inflection point, the tangent, if it exists, intersects the curve, because on one side of this point the curve lies under the tangent, and on the other side - above it.
Let us determine sufficient conditions for the fact that given point the curve is the point of inflection.
Theorem. Let the curve be defined by the equation y = f(x). If f ""(x 0) = 0 or f ""(x 0) does not exist even when passing through the value x = x 0 derivative f ""(x) changes sign, then the point in the graph of the function with the abscissa x = x 0 there is an inflection point.
Proof. Let f ""(x) < 0 при x < x 0 And f ""(x) > 0 at x > x 0. Then at x < x 0 the curve is convex, and when x > x 0– concave. Therefore, the point A, lying on the curve, with abscissa x 0 there is an inflection point. The second case can be considered similarly, when f ""(x) > 0 at x < x 0 And f ""(x) < 0 при x > x 0.
Thus, inflection points should be sought only among those points where the second derivative vanishes or does not exist.
Examples. Find inflection points and determine the intervals of convexity and concavity of curves.
ASYMPTOTES OF THE GRAPH OF THE FUNCTION
When studying a function, it is important to establish the shape of its graph at an unlimited distance of the graph point from the origin.
Of particular interest is the case when the graph of a function, when removed variable point to infinity it approaches a certain straight line without limit.
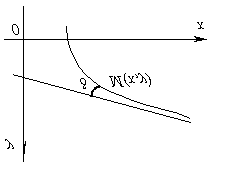
The straight line is called asymptote function graphics y = f(x), if the distance from the variable point M graphics to this line when removing a point M to infinity tends to zero, i.e. a point on the graph of a function, as it tends to infinity, must indefinitely approach the asymptote.
A curve can approach its asymptote while remaining on one side of it or with different sides, infinite set once crossing the asymptote and moving from one side to the other.
If we denote by d the distance from the point M curve to the asymptote, then it is clear that d tends to zero as the point moves away M to infinity.
We will further distinguish between vertical and oblique asymptotes.
VERTICAL ASYMPTOTES
Let at x→ x 0 from any side function y = f(x) increases unlimitedly in absolute value, i.e. or or 
. Then from the definition of an asymptote it follows that the straight line x = x 0 is an asymptote. The opposite is also obvious, if the line x = x 0 is an asymptote, i.e. .
Thus, the vertical asymptote of the graph of the function y = f(x) is called a straight line if f(x)→ ∞ under at least one of the conditions x→ x 0– 0 or x → x 0 + 0, x = x 0
Therefore, to find the vertical asymptotes of the graph of the function y = f(x) need to find those values x = x 0, at which the function goes to infinity (suffers an infinite discontinuity). Then the vertical asymptote has the equation x = x 0.
Examples.
SLANT ASYMPTOTES
Since the asymptote is a straight line, then if the curve y = f(x) has an oblique asymptote, then its equation will be y = kx + b. Our task is to find the coefficients k And b.
Theorem. Straight y = kx + b serves as an oblique asymptote at x→ +∞ for the graph of the function y
= f(x) then and only when 
. A similar statement is true for x → –∞.
Proof. Let MP– length of the segment, equal to the distance from point M to asymptote. According to the condition. Let us denote by φ the angle of inclination of the asymptote to the axis Ox. Then from ΔMNP it follows that . Since φ is a constant angle (φ ≠ π/2), then, but
Instructions
Points inflection functions must belong to the domain of its definition, which must be found first. Schedule functions is a line that can be continuous or have breaks, monotonically decrease or increase, have minimum or maximum points(asymptotes), be convex or concave. Abrupt change of two latest states and is called an inflection.
Prerequisite existence inflection functions consists in the equality of the second to zero. Thus, by differentiating the function twice and equating the resulting expression to zero, we can find the abscissa of possible points inflection.
This condition follows from the definition of the properties of convexity and concavity of the graph functions, i.e. negative and positive value second derivative. At the point inflection abrupt change these properties, which means that the derivative passes the zero mark. However, equal to zero is not yet enough to indicate an inflection.
There are two sufficient conditions that the abscissa found at the previous stage belongs to the point inflection:Through this point you can draw a tangent to functions. The second derivative has different signs to the right and left of the expected one points inflection. Thus, its existence at the point itself is not necessary; it is enough to determine that at it it changes sign. Second derivative functions is equal to zero, and the third is not.
Solution: Find . IN in this case there are no restrictions, therefore, it is the entire space of real numbers. Calculate the first derivative: y’ = 3 ∛(x - 5) + (3 x + 3)/∛(x - 5)².
Please note. It follows from this that the domain of definition of the derivative is limited. The point x = 5 is punctured, which means that a tangent can pass through it, which partly corresponds to the first sign of sufficiency inflection.
Determine the resulting expression for x → 5 – 0 and x → 5 + 0. They are equal to -∞ and +∞. You have proven that a vertical tangent passes through the point x=5. This point may turn out to be a point inflection, but first calculate the second derivative: Y'' = 1/∛(x - 5)² + 3/∛(x - 5)² – 2/3 (3 x + 3)/∛(x - 5)^5 = (2 x – 22)/∛(x - 5)^5.
Omit the denominator since you have already taken into account the point x = 5. Solve the equation 2 x – 22 = 0. It has a single root x = 11. The last step is to confirm that points x=5 and x=11 are points inflection. Analyze the behavior of the second derivative in their vicinity. Obviously, at the point x = 5 it changes sign from “+” to “-”, and at the point x = 11 – vice versa. Conclusion: both points are points inflection. The first sufficient condition is satisfied.
