Imaginary unit- a complex number whose square is equal to negative one.
In mathematics and physics, the imaginary unit is designated as Latin i or j. It allows you to expand the field of real numbers to the field of complex numbers. Precise definition depends on the method of this extension.
The main reason for introducing the imaginary unit is that not every polynomial equation f(x) = 0 with real coefficients has solutions in the field of real numbers. For example, the equation x 2 + 1 = 0 has no real roots. However, if we assume that the roots are complex numbers, then this equation, like any another polynomial equation has a solution.
The statement that the imaginary unit is the “square root of −1” is not entirely correct, because −1 has two arithmetic square roots, one of which can be designated as i, and the other as − i.
Definition
An imaginary unit is a number whose square is equal to −1. Thus i is the solution to the equation
or
If we define i Thus, we will consider it an unknown (“imaginary”, “imaginary”) variable, then the second solution to the equation will be − i, which can be checked by substitution.
Who opened it and when: Italian mathematician Gerolamo Cardano, friend of Leonardo da Vinci, in 1545.
The number i cannot be called a constant or even a real number. Textbooks describe it as a quantity that, when squared, gives minus one. In other words, it is the side of the square with negative area. In reality this does not happen. But sometimes you can also benefit from the unreal.
The history of the discovery of this constant is as follows. The mathematician Gerolamo Cardano, while solving equations with cubes, introduced the imaginary unit. This was just an auxiliary trick - there was no i in the final answers: results that contained it were discarded. But later, having taken a closer look at their “garbage,” mathematicians tried to put it to work: multiplying and dividing regular numbers per imaginary unit, add the results to each other and substitute them into new formulas. This is how the theory of complex numbers was born.
The downside is that “real” cannot be compared with “unreal”: it won’t work to say that the greater is an imaginary unit or 1. On the other hand, there are practically no unsolvable equations left if you use complex numbers. Therefore, with complex calculations, it is more convenient to work with them and only “clean up” the answers at the very end. For example, to decipher a brain tomogram, you cannot do without i.
This is exactly how physicists treat fields and waves. We can even consider that they all exist in a complex space, and that what we see is only a shadow of the “real” processes. Quantum mechanics, where both the atom and the person are waves, makes this interpretation even more convincing.
The number i allows you to summarize the main mathematical constants and actions in one formula. The formula looks like this: eπi+1 = 0, and some say that such a condensed set of rules of mathematics can be sent to aliens to convince them of our intelligence.
Expressions of the form that appear when solving quadratic and cubic equations began to be called “imaginary” in XVI-XVII centuries, however, even for many prominent scientists of the 17th century, the algebraic and geometric essence of imaginary quantities seemed unclear. Leibniz, for example, wrote: “The Spirit of God found the subtlest outlet in this miracle of analysis, a monster from the world of ideas, a dual essence located between being and non-being, which we call the imaginary root of the negative unity.”
For a long time it was unclear whether all operations on complex numbers lead to complex results, or whether, for example, extracting a root could lead to the discovery of some new type of numbers. The problem of expressing roots of degrees n from given number was solved in the works of Moivre (1707) and Cotes (1722).
The symbol was proposed by Euler (1777, published 1794), who took the first letter of the Latin word for this. imaginarius. He spread everything standard features, including the logarithm, onto the complex domain. Euler also expressed the idea in 1751 that the field of complex numbers is algebraically closed. D'Alembert (1747) came to the same conclusion, but the first strict proof This fact belongs to Gauss (1799). Gauss introduced the term “complex number” into widespread use in 1831, although the term had previously been used in the same sense French mathematician Lazare Carnot in 1803.
The geometric interpretation of complex numbers and operations on them first appeared in the work of Wessel (1799). The first steps in this direction were taken by Wallis (England) in 1685. Modern geometric representation, sometimes called the “Argand diagram,” came into use after the publication in 1806 and 1814 of J. R. Argand’s work, which independently repeated Wessel’s conclusions.
Arithmetic model of complex numbers as pairs real numbers was built by Hamilton (1837); this proved the consistency of their properties. Hamilton also proposed a generalization of complex numbers - quaternions, the algebra of which is non-commutative.
Consider the incomplete quadratic equation:
x 2 = a,
Where A is a known quantity. The solution to this equation can be written as:
There are three possible cases here:
| 1). | If a = 0, then x = 0. |
| 2). | If A – positive number, then its square root has two meanings: one positive, the other negative; for example the equation x 2 = 25 has two roots: 5 and – 5. This is often written as a root with a double sign: |
| 3). | If A is a negative number, then this equation has no solutions among the positive and negative numbers known to us, because the second power of any number is the number non-negative. But if we want to obtain solutions to the equation x 2 = a also for negative values A, we are forced to introduce numbers of a new type - imaginary numbers . Thus, imaginary the number is called the second power of which is a negative number. According to this definition of imaginary numbers we can define and imaginary unit: |
Then for the equation x 2 = – 25 we get two imaginary root:
Substituting both of these roots into our equation, we obtain the identity. Unlike imaginary numbers, all other numbers (positive and negative, integers and fractions, rational and irrational) are called valid or real numbers . The sum of a real and an imaginary number is called complex number and is designated:
a + b i ,
Where a, b– real numbers, i– imaginary unit.
For more information about complex numbers, see the section "Complex Numbers".
Examples of complex numbers: 3 + 4 i, 7 – 13.6 i , 0 + 25 i = 25 i , 2 + i.
Powers of imaginary unit
Degrees i repeated in a loop:
Which can be written for any degree in the form:
Where n- any integer.
From here:
Where mod 4 represents the remainder when divided by 4.
The reason for introducing an imaginary unit is that not every polynomial equation with real coefficients has solutions in the field of real numbers. So, the equation has no real roots. However, it turns out that any polynomial equation with complex coefficients has comprehensive solution- “Fundamental theorem of algebra.”
Historically, the imaginary unit was first introduced to solve the real cubic equation: often, if there were three real roots, to obtain two of them, Cardano's formula required taking the cube root in complex numbers.
The statement that the imaginary unit is the “square root of −1” is not precise: after all, “−1” has two square roots, one of which can be designated as “i” and the other as “−i”. It does not matter which root is taken as the imaginary unit: all equalities will remain valid if all “i” are simultaneously replaced with “-i” and “-i” with “i”. However, because of this ambiguity, in order to avoid erroneous calculations, one should not use the notation for through the radical (as ).
Definition
An imaginary unit is a number whose square is −1. Those. is one of the solutions to the equation
or
And then his second solution to the equation will be , which is verified by substitution.
Powers of imaginary unit
Degrees repeated in a loop:
Which can be written for any degree in the form:
Where n- any integer.
From here: Where mod 4 is the remainder of division by 4.
Number is real:
Factorial
Imaginary unit factorial i can be defined as the value of the gamma function from the argument 1 + i :
Roots of imaginary unit
In the field of complex numbers, the root n-th degree has n decisions. On complex plane the roots of the imaginary unit are at the vertices of a regular n-gon inscribed in a circle with unit radius.
This follows from Moivre's formula and the fact that the imaginary unit can be represented in trigonometric form:
In particular, And
Also, roots of an imaginary unit can be represented in exponential form:
Other imaginary units
In the Cayley-Dixon construction (or in Clifford algebras), there can be several “imaginary expansion units”, and/or their square can be ="+1" or even ="0". But in this case, zero divisors may appear, and there are other properties that differ from the properties of complex “i”. For example, there are three anticommutative imaginary units in the quaternion body, and there are also infinitely many solutions to the equation “ ».
On the issue of interpretation and name
| Gauss also argued that if the quantities 1, −1 and √−1 were called, respectively, not positive, negative and imaginary units, but direct, inverse and secondary, then people would not have the impression that there is some kind of connection with these numbers. dark secret. According to Gauss, geometric representation gives the true metaphysics of imaginary numbers in a new light. It was Gauss who introduced the term “complex numbers” (as opposed to Descartes’ “imaginary numbers”) and used the symbol i to denote √−1. Maurice Kline, “Mathematics. Loss of certainty." Chapter VII. Illogical development: serious difficulties on the threshold of the 19th century. |
Designations
Common designation , but in radio engineering the imaginary unit is usually denoted so as not to be confused with the designation of instantaneous current: .
See also
- Dual numbers and Double numbers
Write a review about the article "Imaginary unit"
Notes
Links
- Imaginary unit // Great Soviet Encyclopedia: [in 30 volumes] / ch. ed. A. M. Prokhorov. - 3rd ed. - M. : Soviet encyclopedia, 1969-1978.
|
||||||||||||||||||||||||||
Excerpt characterizing the Imaginary Unit
Several merchants crowded around the officer.- Eh! it's a waste of time to lie! - said one of them, thin, with a stern face. “When you take off your head, you don’t cry over your hair.” Take whatever you like! “And he waved his hand with an energetic gesture and turned sideways to the officer.
“It’s good for you, Ivan Sidorich, to speak,” the first merchant spoke angrily. - You are welcome, your honor.
- What should I say! – the thin man shouted. “I have a hundred thousand goods in three shops here.” Can you save it when the army has left? Eh, people, God's power don't fold it with your hands!
“Please, your honor,” said the first merchant, bowing. The officer stood in bewilderment, and indecision was visible on his face.
- What do I care! - he suddenly shouted and walked with quick steps forward along the row. In one open shop, blows and curses were heard, and while the officer was approaching it, a man in a gray overcoat and with a shaved head jumped out of the door.
This man, bending over, rushed past the merchants and the officer. The officer attacked the soldiers who were in the shop. But at that time, terrible screams of a huge crowd were heard on the Moskvoretsky Bridge, and the officer ran out onto the square.
- What's happened? What's happened? - he asked, but his comrade was already galloping towards the screams, past St. Basil the Blessed. The officer mounted and rode after him. When he approached the bridge, he saw two cannons removed from the limbers, infantry walking along the bridge, several fallen carts, several scared faces and the laughing faces of the soldiers. Near the cannons stood one cart drawn by a pair. Behind the cart, four greyhounds in collars huddled behind the wheels. There was a mountain of things on the cart, and at the very top, next to the children's chair, a woman was sitting with her legs turned upside down, screaming shrilly and desperately. The comrades told the officer that the scream of the crowd and the squeals of the woman occurred because General Ermolov, who drove into this crowd, having learned that the soldiers were scattering among the shops and crowds of residents were blocking the bridge, ordered the guns to be removed from the limbers and an example was made that he would shoot at the bridge . The crowd, knocking down the carts, crushing each other, screaming desperately, crowding in, cleared the bridge, and the troops moved forward.
Meanwhile, the city itself was empty. There was almost no one on the streets. The gates and shops were all locked; here and there near the taverns lonely screams or drunken singing were heard. No one drove along the streets, and pedestrian footsteps were rarely heard. On Povarskaya it was completely quiet and deserted. In the huge courtyard of the Rostovs' house there were scraps of hay and droppings from a transport train, and not a single person was visible. In the Rostov house, which was left with all its good things, two people were in the large living room. These were the janitor Ignat and the Cossack Mishka, Vasilich’s grandson, who remained in Moscow with his grandfather. Mishka opened the clavichord and played it with one finger. The janitor, arms akimbo and smiling joyfully, stood in front of a large mirror.
- That’s clever! A? Uncle Ignat! - the boy said, suddenly starting to clap the keys with both hands.
- Look! - Ignat answered, marveling at how his face smiled more and more in the mirror.
- Shameless! Really, shameless! – the voice of Mavra Kuzminishna, who quietly entered, spoke from behind them. - Eka, thick-horned, he bares his teeth. Take you on this! Everything there is not tidy, Vasilich is knocked off his feet. Give it time!
Ignat, adjusting his belt, stopped smiling and submissively lowered his eyes, walked out of the room.
“Auntie, I’ll go easy,” said the boy.
- I'll give you a light one. Little shooter! – Mavra Kuzminishna shouted, raising her hand at him. - Go and set up a samovar for grandfather.
Mavra Kuzminishna, brushing off the dust, closed the clavichord and, sighing heavily, left the living room and locked the front door.
Coming out into the courtyard, Mavra Kuzminishna thought about where she should go now: should she drink tea in Vasilich’s outbuilding or tidy up what had not yet been tidied up in the pantry?
Quick steps were heard in the quiet street. The steps stopped at the gate; the latch began to knock under the hand that was trying to unlock it.
Mavra Kuzminishna approached the gate.
- Who do you need?
- Count, Count Ilya Andreich Rostov.
- Who are you?
- I'm an officer. “I would like to see,” said the Russian pleasant and lordly voice.
Mavra Kuzminishna unlocked the gate. And a round-faced officer, about eighteen years old, with a face similar to the Rostovs, entered the courtyard.
- We left, father. “We deigned to leave at vespers yesterday,” Mavra Kuzmipishna said affectionately.
The young officer, standing at the gate, as if hesitant to enter or not to enter, clicked his tongue.
“Oh, what a shame!..” he said. - I wish I had yesterday... Oh, what a pity!..
Mavra Kuzminishna, meanwhile, carefully and sympathetically examined the familiar features of the Rostov breed in the face young man, and the tattered overcoat, and the worn-out boots that he was wearing.
- Why did you need a count? – she asked.
- Yeah... what to do! - the officer said with annoyance and grabbed the gate, as if intending to leave. He stopped again, undecided.
– Do you see? - he suddenly said. “I am a relative of the count, and he has always been very kind to me.” So, you see (he looked at his cloak and boots with a kind and cheerful smile), and he was worn out, and there was no money; so I wanted to ask the Count...
Mavra Kuzminishna did not let him finish.
Imaginary unit- usually a complex number whose square is equal to −1 (minus one). However, other options are also possible: in the construction of doubling according to Cayley-Dixon or within the framework of algebra according to Clifford.
For complex numbers
In mathematics and physics, the imaginary unit is designated as Latin texvc or Unable to parse expression (Executable file texvc . It allows you to expand the field of real numbers to the field of complex numbers. The exact definition depends on the extension method.
The reason for introducing an imaginary unit is that not every polynomial equation Unable to parse expression (Executable file texvc not found; See math/README for help with setup.): f(x)=0 with real coefficients has solutions in the field of real numbers. So, the equation Unable to parse expression (Executable file texvc not found; See math/README for setup help.): x^2 + 1 = 0 has no real roots. However, it turns out that any polynomial equation with complex coefficients has a complex solution - the "Fundamental Theorem of Algebra".
Historically, the imaginary unit was first introduced to solve the real cubic equation: often, if there were three real roots, to obtain two of them, Cardano's formula required taking the cube root in complex numbers.
The statement that the imaginary unit is the “square root of −1” is not precise: after all, “−1” has two square roots, one of which can be designated as “i” and the other as “−i”. It does not matter which root is taken as the imaginary unit: all equalities will remain valid if all “i” are simultaneously replaced with “-i” and “-i” with “i”. However, because of this ambiguity, in order to avoid erroneous calculations, one should not use the notation for Unable to parse expression (Executable file texvc not found; See math/README for setup help.): i through the radical (as Unable to parse expression (Executable file texvc not found; See math/README for setup help.): \sqrt(-1)).
Definition
An imaginary unit is a number whose square is −1. Those. Unable to parse expression (Executable file texvc not found; See math/README for setup help.): i is one of the solutions to the equation
texvc not found; See math/README for setup help.): x^2 + 1 = 0, or Unable to parse expression (Executable file texvc not found; See math/README for help with setup.): x^2 = -1.
And then his second solution to the equation will be Unable to parse expression (Executable file texvc not found; See math/README for setup help.): -i, which is verified by substitution.
Powers of imaginary unit
Degrees Unable to parse expression (Executable file texvc not found; See math/README for setup help.): i repeated in a loop:
texvc
Unable to parse expression (Executable file texvc not found; See math/README for help with setup.): i^(-3) = i
Unable to parse expression (Executable file texvc not found; See math/README for help with setup.): i^(-2) = -1
Unable to parse expression (Executable file texvc not found; See math/README for setup help.): i^(-1) = -i
Unable to parse expression (Executable file texvc not found; See math/README - help with setup.): i^0 = 1
Unable to parse expression (Executable file texvc not found; See math/README for setup help.): i^1 = i
Unable to parse expression (Executable file texvc not found; See math/README - help with setup.): i^2 = -1
Unable to parse expression (Executable file texvc not found; See math/README for setup help.): i^3 = -i
Unable to parse expression (Executable file texvc not found; See math/README - help with setup.): i^4 = 1
Unable to parse expression (Executable file texvc not found; See math/README for setup help.): \ldots
Which can be written for any degree in the form:
Unable to parse expression (Executable filetexvc not found; See math/README for setup help.): i^(4n) = 1
Unable to parse expression (Executable file texvc not found; See math/README for help with setup.): i^(4n+1) = i
Unable to parse expression (Executable file texvc not found; See math/README for help with setup.): i^(4n+2) = -1
Unable to parse expression (Executable file texvc not found; See math/README for help on setting up.): i^(4n+3) = -i.
Where n- any integer.
From here: Unable to parse expression (Executable file texvc not found; See math/README for setup help.): i^n = i^(n \bmod 4) Where mod 4 is the remainder of division by 4.
Number Unable to parse expression (Executable file texvc not found; See math/README - help with setup.): i^i is real:
texvc not found; See math/README - help with setup.): i^i=(e^((i\pi/2)i))=e^(i^2\pi/2)=e^(-\pi/ 2)=0(,)20787957635\ldots
Factorial
Imaginary unit factorial i can be defined as the value of the gamma function from the argument 1 + i :
Unable to parse expression (Executable filetexvc not found; See math/README for setup help.): i! = \Gamma(1+i) \approx 0.4980 - 0.1549i.
Unable to parse expression (Executable file texvc not found; See math/README for setup help.): |i!| = \sqrt(\pi \over \sinh(\pi)) \approx 0.521564... .
Roots of imaginary unit
In the field of complex numbers, the root n-th degree has n decisions. In the complex plane, the roots of the imaginary unit are located at the vertices of a regular n-gon inscribed in a circle with unit radius.
Unable to parse expression (Executable filetexvc not found; See math/README for help on setting up.): u_k=\cos (\frac((\frac(\pi)(2)) + 2\pi k)(n)) +i\ \sin (\frac( (\frac(\pi)(2)) + 2\pi k)(n)), \quad k=0,1,...,n-1
This follows from Moivre's formula and the fact that the imaginary unit can be represented in trigonometric form:
Unable to parse expression (Executable filetexvc not found; See math/README - help with setup.): i=\cos\ (\frac(\pi)(2)) + i\ \sin\ (\frac(\pi)(2))
In particular, Unable to parse expression (Executable file texvc not found; See math/README for help with setup.): \sqrt(i ) = \left\(\frac(1 + i)(\sqrt(2));\ \frac(-1 - i)(\sqrt( 2)) \right\) And Unable to parse expression (Executable file texvc not found; See math/README for setup help.): \sqrt(i ) = \left\(-i;\ \frac(i + (\sqrt(3)))(2);\ \frac(i - ( \sqrt(3)))(2) \right\)
Also, roots of an imaginary unit can be represented in exponential form:
Unable to parse expression (Executable filetexvc not found; See math/README - help with setup.): u_k=e^(\frac((\frac(\pi)(2) + 2\pi k) i)(n) ), \quad k=0,1 ,...,n-1
Other imaginary units
In the Cayley-Dixon construction (or in Clifford algebras), there can be several “imaginary expansion units”, and/or their square can be ="+1" or even ="0". But in this case, zero divisors may appear, and there are other properties that differ from the properties of complex “i”. For example, there are three anticommutative imaginary units in the quaternion body, and there are also infinitely many solutions to the equation “ Unable to parse expression (Executable file texvc not found; See math/README for setup help.): x^2 = -1
».
On the issue of interpretation and name
| Gauss also argued that if the quantities 1, −1 and √−1 were called, respectively, not positive, negative and imaginary units, but direct, inverse and secondary, then people would not have the impression that some kind of gloomy thing is associated with these numbers secret. According to Gauss, geometric representation gives the true metaphysics of imaginary numbers in a new light. It was Gauss who introduced the term “complex numbers” (as opposed to Descartes’ “imaginary numbers”) and used the symbol i to denote √−1. Maurice Kline, “Mathematics. Loss of certainty." Chapter VII. Illogical development: serious difficulties on the threshold of the 19th century. |
Designations
Common designation Unable to parse expression (Executable file texvc not found; See math/README for setup help.): i, but in radio engineering the imaginary unit is usually denoted Unable to parse expression (Executable file texvc not found; See math/README for setup help.): j, so as not to be confused with the designation of instantaneous current: Unable to parse expression (Executable file texvc not found; See math/README for setup help.): i = i (t)
.
See also
- Dual numbers and Double numbers
Write a review about the article "Imaginary unit"
Notes
Links
- Imaginary unit // Great Soviet Encyclopedia: [in 30 volumes] / ch. ed. A. M. Prokhorov. - 3rd ed. - M. : Soviet Encyclopedia, 1969-1978.
|
||||||||||||||||||||||||||
Excerpt characterizing the Imaginary Unit
– This cannot be taught, Isidora. People must have a need for Light, a need for Good. They themselves must want change. For what is given by force, a person instinctively tries to quickly reject, without even trying to understand anything. But we digress, Isidora. Do you want me to continue the story of Radomir and Magdalena?I nodded affirmatively, deeply regretting in my heart that I couldn’t have a conversation with him so simply and calmly, without worrying about what fate had given me last minutes my crippled life and not thinking with horror about the misfortune hanging over Anna...
– The Bible writes a lot about John the Baptist. Was he truly with Radomir and the Knights of the Temple? His image is so amazingly good that it sometimes made one doubt whether John was the real figure? Can you answer, North?
North smiled warmly, apparently remembering something very pleasant and dear to him...
– John was wise and kind, like a great warm sun... He was a father to everyone who walked with him, their teacher and friend... He was valued, obeyed and loved. But he was never the young and amazingly handsome young man that artists usually painted him as. John at that time was already an elderly sorcerer, but still very strong and persistent. Gray-haired and tall, he looked more like a mighty epic warrior than an amazingly handsome and gentle young man. He wore very long hair, as well as everyone else who is with Radomir.
It was Radan, he was truly extraordinarily handsome. He, like Radomir, lived in Meteora from an early age, next to his mother, Sorceress Maria. Remember, Isidora, how many paintings there are in which Mary is painted with two, almost the same age, babies. For some reason, all the famous artists painted them, perhaps without even understanding WHO their brush really depicted... And what is most interesting is that it is Radan that Maria looks at in all these paintings. Apparently even then, while still a baby, Radan was already as cheerful and attractive as he remained throughout his short life...
And yet... even if the artists painted John in these paintings, then how could that same John have aged so monstrously by the time of his execution, carried out at the request of the capricious Salome?.. After all, according to the Bible, this happened even before the crucifixion Christ, which means John should have been no more than thirty at that time four years! How did he turn from a girlishly handsome, golden-haired young man into an old and completely unattractive Jew?!
- So the Magus John did not die, Sever? – I asked joyfully. – Or did he die in another way?..
“Unfortunately, the real John really had his head cut off, Isidora, but this did not happen due to the evil will of a capricious spoiled woman. The cause of his death was the betrayal of a Jewish “friend” whom he trusted and in whose house he lived for several years...
- But how come he didn’t feel it? How did you not see what kind of “friend” this was?! – I was indignant.
– It’s probably impossible to suspect every person, Isidora... I think it was already difficult enough for them to trust someone, because they all had to somehow adapt and live in that foreign, unfamiliar country, don’t forget that. Because, from the big and lesser evil they apparently tried to choose less. But it’s impossible to predict everything, you know this very well, Isidora... The death of Magus John occurred after the crucifixion of Radomir. He was poisoned by a Jew, in whose house John was living at that time along with the family of the deceased Jesus. One evening, when the whole house was already asleep, the owner, talking with John, presented him with his favorite tea mixed with a strong herbal poison... The next morning, no one was even able to understand what had happened. According to the owner, John simply instantly fell asleep, and never woke up again... His body was found in the morning in his bloody bed with... a severed head... According to the same owner, the Jews were very afraid of John, because they considered him an unsurpassed magician. And to be sure that he would never rise again, they beheaded him. John’s head was later bought (!!!) from them and taken with them by the Knights of the Temple, managing to preserve it and bring it to the Valley of the Magi, in order to thus give John at least such a small, but worthy and deserved respect, without allowing the Jews to simply mock him, doing some of his own magical rituals. From then on, John's head was always with them, wherever they were. And for this same head, two hundred years later, the Knights of the Temple were accused of criminal worship of the Devil... You remember the last “case of the Templars” (Knights of the Temple), don’t you, Isidora? It was there that they were accused of worshiping a “talking head”, which infuriated the entire church clergy.
- Forgive me, Sever, but why didn’t the Knights of the Temple bring John’s head here to Meteora? Because, as far as I understand, you all loved him very much! And how do you know all these details? You weren't with them, were you? Who told you all this?
- Told us all this sad story Witch Maria, mother of Radan and Radomir...
– Did Mary return to you after the execution of Jesus?!.. After all, as far as I know, she was with her son during the crucifixion. When did she return to you? Is it possible that she is still alive?.. – I asked with bated breath.
Subject: Imaginary unit , her degrees. Complex numbers.
Algebraic form comprehensive numbers.
Goals: expand the concept of number, introduce the concept of an imaginary unit and its powers, the concept complex number; consider the algebraic form of a complex number; develop the ability to generalize acquired knowledge, promote the development logical thinking;
educate students conscious attitude to the learning process.
Plan ( issues being studied )
Imaginary numbers. Definition of an imaginary unit. Powers of the imaginary unit.
Definition of a complex number.
Algebraic form complex number.
1.Imaginary numbers
Definition. A number whose square is -1 is called an imaginary unit and
denoted by і ; і 2 = -1
Definition. Numbers that have the form b і , where b is a real number, are called
imaginary numbers.
For example:
It is known that real numbers are represented by points on the OX axis. Imaginary numbers are represented by points on the OU axis, and therefore the OX axis is called real axis, and the axis OU is the imaginary axis. The set of imaginary numbers is in one-to-one correspondence with the set of real numbers.
Definition. Two imaginary numbers b 1 i And b 2 i are called equal if b 1 = b 2
Definition. Imaginary number (- bi ) called the opposite of an imaginary number b і .
For example:
 And
And

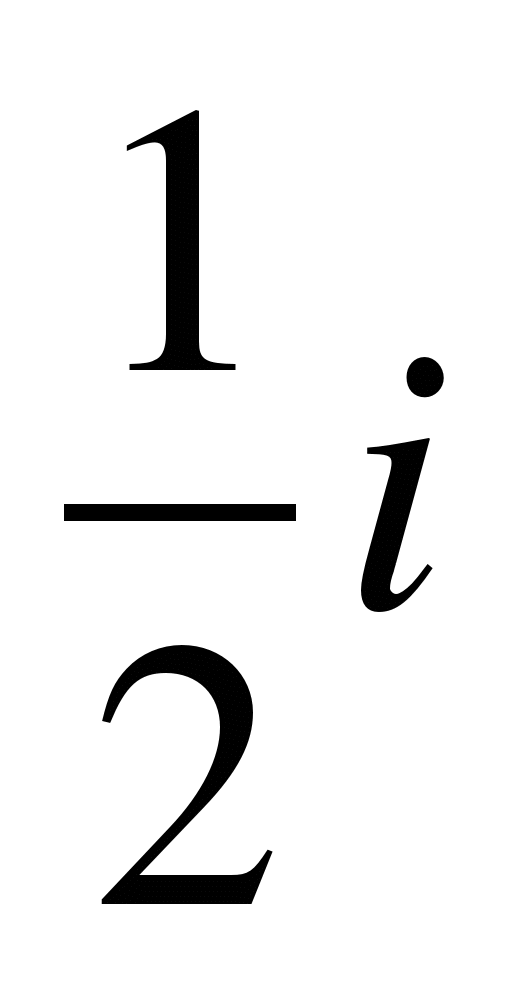 And
And  .
.
Theorem. Any natural power of a number і can be converted to
one of four types 1; і ; -1; -і.
Proof .
Consider the expression і m , where m - natural number. It is clear that four cases are possible:
1) m = 4 k , k =1,2, ...
2) m=4k +1,k =0, 1,2,...
3) m 4k +2, k = 0,1,2,...
4) m = 4k +3, k =0,1,2, ....
Let m = 4 k , Then і m =і Ak =(і A ) To =1 To =1
Letm =4 k +1, Then і m = і Ak+1 = і Ak i=1i=i
Let m = 4 k +2, Thenі m =і Ak+2 = і Ak і 2 = 1(-1)=-1
Letm =4 k +3, Then і m
Example. Calculate the value of an expression
Solution:
Comment. In order to calculate the power of an imaginary unit, it is convenient to use the following rule:
1) divide the exponent by 4;
2) replace i m on i R , where p is the remainder obtained by dividing t by 4, that is, the number p is found from the equality t = 4k + p.
2. Complex numbers
Definition. A complex number is a number that has the forma+bi , where a, b –
real numbers, i is the imaginary unit. In this case, the number “a” is called
real part of a complex number, "b" - imaginary part
complex number.
Symbolically, the real and imaginary parts of a complex number are denoted as follows:(reset), (they don't).
These designations are based on the first letters Latin words, which means "real" and "Imaginaries", which means "imaginary".
Comment. Sometimes the imaginary part of a complex numberz = A + b і called bi.
Definition. Two complex numbersZ 1 = a 1 + b 1 i Andz 2 = A 2 + b 1 i are called equal if
Rez 1 = Rez 2 , Imz 1 = Imz 2 .
For complex numbers there are no concepts of greater and less, that is, complex numbers are not comparable.
Definition. Complex number(-A- bi ) called the opposite of a complex number
a + b.
Definition. Two complex numbers whose real parts are equal and whose imaginary parts are equal
partsopposites are called complex conjugate numbers and
are designated accordingly And.
3. Algebraic form of a complex number. Actions on complex numbers given in algebraic form.
Complex number represented as  called a complex number inalgebraic form
.
called a complex number inalgebraic form
.
Addition of complex numbers
Definition.
The sum of two complex numbers  And
And  called
called
complex number .
So, (1)
Thus, to add two complex numbers, you need to add their real parts, and this gives the real part of the sum, and add the imaginary parts, which gives the imaginary part of the sum.
The sum of conjugate numbers is always real th number
that is,  . (2)
. (2)
Subtracting Complex Numbers
Definition.
The difference of two complex numbers  And
And  this is called
this is called
complex number  , which adds up to the number
, which adds up to the number  gives a number
gives a number  .
.
Subtracting complex numbers is always possible.
Theorem.
For any complex numbers  And
And  there is always a difference
there is always a difference  , which is uniquely determined.
, which is uniquely determined.
Thus, in order to subtract complex numbers, it is enough to subtract their real parts and take their difference as the real part of the difference, and also subtract the imaginary part of the difference
It turns out, (3)
The difference of two conjugate numbers is always an imaginary number. ,
that is,  (4)
(4)
Multiplying complex numbers
Definition.
Product of two complex numbers
 and such a complex number is called, which is determined by the formula: (5)
and such a complex number is called, which is determined by the formula: (5)
To multiply complex numbers, you should multiply them according to the rule for multiplying polynomials, replacing  by -1 and bring similar terms.
by -1 and bring similar terms.
When multiplying complex numbers, it is better to perform direct multiplication. The product of conjugate numbers is always real number Answer.
1.Give the definition of a complex number.
2.Formulate the definition of an imaginary unit.
3. How to find the degree of an imaginary unit.
4.What complex numbers are called equal and conjugate?
5.Write a formula for finding an arbitrary power of an imaginary unit.
6. Give examples of purely imaginary numbers.
7. Define the sum, product and quotient of two complex numbers.
Literature
Written, D. T. Lecture notes on higher mathematics: full course D.T. Written. – 9th ed. – M.: Iris-press, 2009. 608 p.: ill. - (Higher education).
Lungu, K. N. Collection of problems in higher mathematics. 1st year / K. N. Lungu, D. T. Pismenny, S. N. Fedin, Yu. A. Shevchenko. – 7th ed. – M.: Iris-press, 2008. 576 pp.: – (Higher education).
Grigoriev V.P. Elements of higher mathematics: a textbook for students. institutions prof. education / V. P. Grigoriev, Yu. A. Dubinsky. – 10th ed., erased. – M. Publishing center “Academy”, 2014. – 320 p.








